Physics:Methane clumped isotopes

From HandWiki
Short description: Methane molecules that contain two or more rare isotopes

Methane clumped isotopes are methane molecules that contain two or more rare isotopes. Methane (CH4) contains two elements, carbon and hydrogen, each of which has two stable isotopes. For carbon, 98.9% are in the form of carbon-12 (12C) and 1.1% are carbon-13 (13C); while for hydrogen, 99.99% are in the form of protium (1H) and 0.01% are deuterium (2H or D). Carbon-13 (13C) and deuterium (2H or D) are rare isotopes in methane molecules. The abundance of the clumped isotopes provides information independent from the traditional carbon or hydrogen isotope composition of methane molecules.

Introduction

Isotopologues are molecules that have the same chemical composition, but differ only in their isotopic composition. Methane has ten stable isotopologues: 12CH4, 13CH4, 12CH3D, 13CH3D, 12CH2D2, 13CH2D2, 12CHD3, 13CHD3, 12CD4 and 13CD4, among which, 12CH4 is an unsubstituted isotopologue; 13CH4 and 12CH3D are singly substituted isotopologues; 13CH3D and 12CH2D2 are doubly substituted isotopologues. The multiple-substituted isotopologues are clumped isotopologues.

The absolute abundance of each isotopologue primarily depends on the traditional carbon and hydrogen isotope compositions (δ13C and δD) of the molecules. Clumped isotope composition is calculated relative to the random distribution of carbon and hydrogen isotopes in the methane molecules. The deviations from the random distribution is the key signature of methane clumped isotope (please see "notation" for details).

In thermodynamic equilibrium, methane clumped isotopologue composition has a monotonic relationship with formation temperature.[1][2] This is the condition for many geological environments[3] so that methane clumped isotope can record its formation temperature, and therefore can be used to identify the origins of methane. When methane clumped-isotope composition is controlled by kinetic effects, for example, for microbial methane, it has the potential to be used to study metabolism.[4][5]

The study of methane clumped isotopologues is very recent. The first mass spectrometry measurement of methane clumped isotopologues of natural abundance was made in 2014.[2] This is a very young and fast-growing field.

Abundances of isotopologues of methane[2]
Isotopologue Type of Isotopologue Abundance
12CH4 Unsubstituted isotopologue 98.88%
13CH4 Singly-substituted isotopologue 1.07%
12CH3D Singly-substituted isotopologue 0.045%
13CH3D Doubly-substituted isotopologue 0.000492%
12CH2D2 Doubly-substituted isotopologue 7.848×10−6%
13CH2D2 Triply-substituted isotopologue 8.488×10−8%
12CHD3 Triply-substituted isotopologue 6.018×10−10%
13CHD3 Quadruply-substituted isotopologue 6.509×10−12%
12CD4 Quadruply-substituted isotopologue 1.73×10−14%
13CD4 Fully-substituted isotopologue 1.871×10−16%

Assuming isotopes are randomly distributed throughout all isotopologues and isotopes are of natural abundance.

Notation

Δ notation

The Δ notation of clumped isotopes is an analogue to δ notation of traditional isotopes (e.g. δ13C, δ18O, δ15N, δ34S and δD).

The notation of traditional isotopes are defined as:

[math]\displaystyle{ \delta=(\left ( \frac{R_{sample}}{R_{reference}} \right )-1)\times1000 }[/math]‰

[math]\displaystyle{ R_{sample} }[/math] is the ratio of the rare isotope to the abundant isotope in the sample. [math]\displaystyle{ R_{reference} }[/math] is the same ratio in the reference material. Because the variation of [math]\displaystyle{ R_{sample} }[/math] is rather small, in the convenience of comparison between difference samples, the notation is define as a ratio minus 1 and expressed in permil (‰).

The Δ notation is inherited from traditional δ notation. But the reference is not a physical reference material. Instead, the reference frame is defined as the stochastic distribution of isotopologues in the sample. It means the values of Δ are to denote the excess or deficit of the isotopologue relative to the amount expected if a material conforms to the stochastic distribution.[6]

The calculation of stochastic distribution of methane isotopologues:

[math]\displaystyle{ ^{^{13}CH_3D}R^*= 4\times{^2R}\times{^{13}R} }[/math]

[math]\displaystyle{ ^{^{12}CH_2D_2}R^*= 6\times{^2R}^2 }[/math]

where [math]\displaystyle{ ^{^{13}CH_3D}R^* }[/math] is defined as the abundance of 13CH3D molecules relative to 12CH4 molecules in random distribution; [math]\displaystyle{ ^{^{12}CH_2D_2}R^* }[/math] is defined as the abundance of 12CH2D2 molecules relative to 12CH4 molecules in random distribution; [math]\displaystyle{ {^2R}=\frac{[D]}{[H]} }[/math] calculates the abundance of deuterium relative to protium in all methane molecules; [math]\displaystyle{ {^{13}R}=\frac{[^{13}C]}{[^{12}C]} }[/math] calculates the abundance of carbon-13 relative to carbon-12 in all methane molecules.

For the random distribution (i.e. probability distribution), the probability of choosing a carbon-13 atom over a carbon-12 atom is [math]\displaystyle{ {^{13}R} }[/math]; the probability of choosing three protium atoms and one deuterium atom over four protium atoms is[math]\displaystyle{ \binom 41 \times{^2R} }[/math] (see "Combination") . Therefore, the probability of the occurrence of a 13CH3D molecule relative to the occurrence of a 12CH4 molecule is the product of [math]\displaystyle{ {^{13}R} }[/math] and [math]\displaystyle{ 4\times{^2R} }[/math], which gets to [math]\displaystyle{ ^{^{13}CH_3D}R^*= 4\times{^2R}\times{^{13}R} }[/math]. Similarly, the probability of choosing two protium atoms and two deuterium atoms over four protium atoms is [math]\displaystyle{ \binom 42\times{^2R}^2 }[/math]. Therefore, the probability of the occurrence of a 12CH2D2 molecule relative to the occurrence of a 12CH4 molecule is [math]\displaystyle{ \binom 42\times{^2R}^2 }[/math], which gets to [math]\displaystyle{ ^{^{12}CH_2D_2}R^*= 6\times{^2R}^2 }[/math].

The calculation of deviation from the random distribution:

[math]\displaystyle{ \Delta_{^{13}CH_3D}=\left ( \frac{^{^{13}CH_3D}R}{^{^{13}CH_3D}R^*} \right )-1 }[/math]

[math]\displaystyle{ \Delta_{{}^{12}CH_2D_2}=\left ( \frac{^{^{12}CH_2D_2}R}{^{^{12}CH_2D_2}R^*} \right )-1 }[/math]

where the actual abundance of 13CH3D molecules relative to 12CH4 molecules, and the actual abundance of 12CH2D2 molecules relative to 12CH4 molecules are calculated as follows:

[math]\displaystyle{ ^{^{13}CH_3D}R= \frac{[^{13}CH_3D]}{[^{12}CH_4]} }[/math]

[math]\displaystyle{ ^{^{12}CH_2D_2}R= \frac{[^{12}CH_2D_2]}{[^{12}CH_4]} }[/math]

The two Δ formulas are frequently used to report the abundance of clumped isotopologues of methane.

The reason for choosing stochastic distribution as the reference frame may be historical - in the process of developing CO2 clumped isotope measurement, the only material with known clumped isotope abundance was CO2 heated to 1000 °C. However, this reference frame is a good choice. Because the absolute abundance of each isotopologue primarily depends on the bulk carbon and hydrogen isotope compositions (δ13C and δD) of the molecules, i.e. very close to stochastic distribution. Therefore, the deviation from the stochastic distribution, which is the key information embedded in the methane clumped isotopologues, is denoted by Δ values.

Mass-18 notation

Under some circumstances, the abundances of 13CH3D and 12CH2D2 isotopologues are only measured as a sum, which leads to the notation for isotopologues of mass-18 (i.e. 13CH3D and 12CH2D2):

[math]\displaystyle{ ^{18}R= ^{^{13}CH_3D}R+^{^{12}CH_2D_2}R=\frac{[^{13}CH_3D]+[^{12}CH_2D_2]}{[^{12}CH_4]} }[/math]

[math]\displaystyle{ \Delta_{18}=\left ( \frac{^{18}R}{^{18}R^*} \right )-1 }[/math]

Note that [math]\displaystyle{ \Delta_{18} }[/math] is not just the sum of [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] and [math]\displaystyle{ \Delta_{{}^{12}CH_2D_2} }[/math].

Inferred equilibration temperature

[math]\displaystyle{ T_{18} }[/math] is the inferred equilibration temperature based on [math]\displaystyle{ \Delta_{18} }[/math] values; [math]\displaystyle{ T_{^{13}CH_3D} }[/math] is the inferred equilibration temperature based on [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values; and [math]\displaystyle{ T_{^{12}CH_2D_2} }[/math] is the inferred equilibration temperature based on [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math] values (see "Equilibrium thermodynamics" for details). [math]\displaystyle{ T_{18} }[/math], [math]\displaystyle{ T_{^{13}CH_3D} }[/math], and [math]\displaystyle{ T_{^{12}CH_2D_2} }[/math] are also called clumped-isotope temperatures. When a Δ value is smaller than zero, there is no inferred equilibration temperature associated with it. Because at any finite temperature, the equilibrium Δ value is always positive.

Physical chemistry

Equilibrium thermodynamics

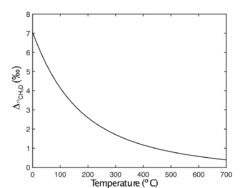
When formed or re-equilibrated in reversible reactions, methane molecules can exchange isotopes with each other or with other substances present, such as H2O, H2 and CO2,[4] and reach internal isotopic equilibrium. As a result, clumped isotopologues are enriched relative to the stochastic distribution. [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values of methane in internal isotopic equilibrium are predicted[1][7][8][2][9] and verified[10][9] to vary as monotonic functions of temperature of equilibration as follows:

[math]\displaystyle{ \Delta_{18}=-0.0117{\left ( \frac{10^6}{T^2} \right )}^2+0.708\left ( \frac{10^6}{T^2} \right )-0.337 }[/math][2]

[math]\displaystyle{ \Delta_{^{13}CH_3D}=-0.0141{\left ( \frac{10^6}{T^2} \right )}^2+0.699\left ( \frac{10^6}{T^2} \right )-0.311 }[/math][11]

The equilibrium distribution of Δ18 as a monotonic function of temperature. Redrawn from Stolper et al., 2014.

Δ values are in permil (‰).

The equilibrium distribution of Δ13CH3D as a monotonic function of temperature. Redrawn from Webb and Miller, 2014.

Similar relationship also applies to [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math]:

The equilibrium distribution of [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math] as a monotonic function of temperature. Redrawn from Young et al., 2017.

[math]\displaystyle{ \Delta_{^{12}CH_2D_2}={\left ( \frac{0.183798}T \right )}-{\left ( \frac{785.483}{T^2} \right )}+\left ( \frac{1056280.0}{T^3} \right )+\left ( \frac{9.37307\times10^7}{T^4} \right ) -\left ( \frac{8.919480\times10^{10}}{T^5} \right )+\left ( \frac{9.901730\times10^{12}}{T^6} \right ) }[/math][12]

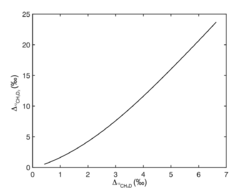
The equilibrium distribution of [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math]. Redrawn from Young et al., 2017.

Based on these correlations, [math]\displaystyle{ \Delta_{18} }[/math], [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] and [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math] can be used as a geothermometer to indicate the formation temperature of methane ([math]\displaystyle{ T_{18} }[/math], [math]\displaystyle{ T_{^{13}CH_3D} }[/math] and [math]\displaystyle{ T_{^{12}CH_2D_2} }[/math]). And the correlation of [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] and [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math]can help to determine whether methane is formed in internal isotopic equilibrium.[12]

Kinetic isotope effects

Kinetic isotope effect (KIE) occurs in irreversible reactions, such as methanogenesis, and can deviate methane clumped isotopologue composition from its thermodynamic equilibrium. Normally, KIE significantly drives [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] lower than their equilibrium states and even to negative values (i.e. more depleted of clumped isotopologues than stochastic distribution.[9][13][14][12][5] Such lower [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values correspond to apparent formation temperatures that are significantly higher than actual formation temperature, or to no possible temperatures (when a Δ value is smaller than zero, there is no inferred equilibration temperature associated with it).

Mixing effect

Mixing between end-members with different conventional carbon and hydrogen isotope compositions (i.e. δ13C, δD) results in non-linear variations in [math]\displaystyle{ \Delta_{18} }[/math] or [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math]. This non-linearity results from the non-linear definition of [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values in reference to the random distributions of methane isotopologues ([math]\displaystyle{ ^{^{13}CH_3D}R^*= 4\times{^2R}\times{^{13}R} }[/math] and [math]\displaystyle{ ^{18}R^*= ^{^{13}CH_3D}R^*+^{^{12}CH_2D_2}R^*=4\times{^2R}\times{^{13}R}+6\times{^2R}^2 }[/math], as in "Notation"), which are non-linear polynomial functions of δD and δ13C values. Such non-linearity can be a diagnostic signature for mixing if multiple samples of various mixing ratios can be measured. When end-members have similar δ13C or δD compositions, the non-linearity is negligible.[4]

Examples of mixing effects for [math]\displaystyle{ \Delta_{18} }[/math] values. Mixing relationships in δ13C-[math]\displaystyle{ \Delta_{18} }[/math] space and δD-[math]\displaystyle{ \Delta_{18} }[/math] space for mixtures of methane with varying end-member compositions. The end-member [math]\displaystyle{ \Delta_{18} }[/math] values remain fixed, but the end-member δ13C and δD values vary. Redrawn from Douglas et al., 2017.

Measurement techniques

Mass spectrometry

On an isotope-ratio mass spectrometer, the measurement of clumped isotopologues has to be conducted on intact methane molecules, instead of converting methane to CO2, H2 or H2O. High mass resolution is required to distinguish different isotopologues of very close relative molecular mass (same "cardinal mass", e.g. 13CH4 and 12CH3D (17.03465 Da (daltons) versus 17.03758 Da), 13CH3D and 12CH2D2 (18.04093 Da versus 18.04385 Da). Currently, two commercial models capable of such measurement are Thermo Scientific 253 Ultra[15] and the Panorama by Nu Instruments.[16]

Infrared spectroscopy

Tunable infrared laser direct absorption spectroscopy (TILDAS) has been developed to measure the abundance of 13CH3D with two continuous wave quantum cascade lasers.[17]

Theoretical studies

There have been several theoretical studies on equilibrium thermodynamics of methane clumped isotopologues since 2008. These studies are based on ab initio, from underlying physical chemistry principles, and do not rely on empirical, or lab-based, data.

Ma et al. utilized first-principle quantum mechanism molecular calculation (Density Functional Theory, or DFT) to study the temperature dependence of the 13CH3D abundance.[1] Cao and Liu estimated [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] based on statistical mechanics.[7] Webb and Miller combined path-integral Monte Carlo methods with high-quality potential energy surfaces to more rigorously compute equilibrium isotope effects of [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] compared to Urey model using reduced partition function ratios.[11] Piasecki et al. performed first-principles calculations of the equilibrium distributions of all substituted isotopologues of methane.[8]

The overall conclusion of theoretical studies is [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] and [math]\displaystyle{ \Delta_{18} }[/math]vary as decreasing monotonic functions of temperature, and the enrichment of multiply D-substituted > multiply 13C-D-substituted > multiply 13C-substituted isotopologues for a same number of substitutions (as shown in this figure).

The theoretical equilibrium distribution of all singly and multiply substituted isotopologues of methane as a function of temperature, assuming isotopes are of natural abundance. Redrawn from Piasecki et al., 2016.

Distribution in nature

Geosphere

Many studies have observed composition of thermogenic methane in equilibria.[10][13][12] The reported [math]\displaystyle{ T_{18} }[/math] and [math]\displaystyle{ T_{^{13}CH_3D} }[/math] are normally distributed within the range of 72 to 298 °C (peak value: [math]\displaystyle{ 175\pm47 }[/math]°C), which aligns well with modeled results of methane formation temperature and yield.[3] However, some thermogenic methane samples have clumped-isotope temperatures that are unrealistically high.[10][3] Possible explanations for exceedingly high clumped isotope temperatures include natural gas migration after formation, mixing effect, and kinetic isotope effect of secondary cracking.

Biosphere

Methanogenesis is a form of anaerobic respiration used by microbes, and microbial methanogenesis can occur in deep subsurface, marine sediments, freshwater bodies, etc. It appears that methane from deep subsurface and marine sediment is generally in internal isotopic equilibrium.,[10][18][13][14] while freshwater microbial methanogenesis expresses large kinetic isotope effect on methane clumped isotope composition.[13][9][14][12][5]

There are two possible explanations for this variance: firstly, substrate limitation may enhance the reversibility of methanogenesis, thus allowing methane to achieve internal isotopic equilibrium via rapid hydrogen exchange with water;[13][9] secondly, activation of C-H bonds during anaerobic oxidation precedes reversibly such that C-H bonds are broken and reformed faster than the net rate of methane consumption and methane can be reequilibrated.[13]

Experimental studies

Calibration of equilibrium thermodynamics

Theoretical calculations have predicted [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values of methane in internal isotopic equilibrium.[1][7][8][2][9] As there are assumptions and approximations in calculations, the equilibrium distribution is only experimentally validated after the analysis of samples brought to thermodynamic equilibrium.[10][9] Nickel and platinum catalysts have been used to equilibrate methane C-H bonds at various temperatures from 150 to 500 °C in laboratory.[17][2][9][14] Currently, catalytic equilibration is also the practice to develop the reference material for clumped isotope analysis .

Microbial culture

Hydrogenotrophic methanogens utilize CO2 and H2 to produce methane by the following reaction:

CO2 + 4H2 → CH4 + 2H2O

Acetoclastic methanogens metabolize acetate acid and produce methane:

CH3COOH → CH4 + CO2

In laboratories, clumped isotope compositions of methane generated by hydrogenotrophic methanogens,[10][9][12][5] acetoclastic methanogens (biodegradation of acetate),[14][12][5] and methylotrophic methanogens[5] are universally out of equilibria. It has been proposed that the reversibility of methanogenic enzyme is key to the kinetic isotope effect expressed in biogenic methane.[13][9]

Pyrolysis of larger organic molecules

Both pyrolysis of propane and closed-system hydrous pyrolysis of organic matter generate methane of [math]\displaystyle{ T_{18} }[/math] consistent with experimental temperatures.[10] Closed-system nonhydrous pyrolysis of coal yields non-equilibrium distribution of methane isotopologues.[19]

Sabatier reaction

Methane synthesized by Sabatier reaction is largely depleted in CH2D2 and slightly depleted in 13CH3D relative to the equilibrium state. It has been proposed that quantum tunneling effects result in the low [math]\displaystyle{ \Delta_{^{12}CH_2D_2} }[/math] observed in the experiment.[12]

Applications

Distinguishing origins of natural gas

Biogenic, thermogenic and abiotic methane is formed at different temperatures, which can be recorded in clumped isotope compositions of methane.[10][13][14][20][21] Combined with conventional carbon and hydrogen isotope fingerprints and gas wetness (the abundance of low molecular weight hydrocarbon),[22] methane clumped isotope can be used to identify the origins of methane in different types of natural gas accumulations.[3]

Biogeochemistry of microbial methane

In freshwater environments, significant kinetic isotope effect leads to a wide range of observed [math]\displaystyle{ \Delta_{18} }[/math] and [math]\displaystyle{ \Delta_{^{13}CH_3D} }[/math] values, which has the potential to provide insights into methanogenesis rate and chemical condition in the corresponding environments.[4][5]

See also

References

  1. ↑ 1.0 1.1 1.2 1.3 Ma, Qisheng; Wu, Sheng; Tang, Yongchun (November 2008). "Formation and abundance of doubly-substituted methane isotopologues (13CH3D) in natural gas systems". Geochimica et Cosmochimica Acta 72 (22): 5446–5456. doi:10.1016/j.gca.2008.08.014. ISSN 0016-7037. 
  2. ↑ 2.0 2.1 2.2 2.3 2.4 2.5 2.6 Stolper, D.A.; Sessions, A.L.; Ferreira, A.A.; Santos Neto, E.V.; Schimmelmann, A.; Shusta, S.S.; Valentine, D.L.; Eiler, J.M. (February 2014). "Combined 13C–D and D–D clumping in methane: Methods and preliminary results". Geochimica et Cosmochimica Acta 126: 169–191. doi:10.1016/j.gca.2013.10.045. ISSN 0016-7037. 
  3. ↑ 3.0 3.1 3.2 3.3 Stolper, Daniel A.; Lawson, Michael; Formolo, Michael J.; Davis, Cara L.; Douglas, Peter M. J.; Eiler, John M. (2018-01-01). "The utility of methane clumped isotopes to constrain the origins of methane in natural gas accumulations" (in en). Geological Society, London, Special Publications 468 (1): 23–52. doi:10.1144/SP468.3. ISSN 0305-8719. http://sp.lyellcollection.org/content/468/1/23. 
  4. ↑ 4.0 4.1 4.2 4.3 Douglas, Peter M.J.; Stolper, Daniel A.; Eiler, John M.; Sessions, Alex L.; Lawson, Michael; Shuai, Yanhua; Bishop, Andrew; Podlaha, Olaf G. et al. (November 2017). "Methane clumped isotopes: Progress and potential for a new isotopic tracer". Organic Geochemistry 113: 262–282. doi:10.1016/j.orggeochem.2017.07.016. ISSN 0146-6380. http://www.escholarship.org/uc/item/3vk8g0tb. 
  5. ↑ 5.0 5.1 5.2 5.3 5.4 5.5 5.6 Gruen, Danielle S.; Wang, David T.; Könneke, Martin; Topçuoğlu, Begüm D.; Stewart, Lucy C.; Goldhammer, Tobias; Holden, James F.; Hinrichs, Kai-Uwe et al. (2018-09-15). "Experimental investigation on the controls of clumped isotopologue and hydrogen isotope ratios in microbial methane". Geochimica et Cosmochimica Acta 237: 339–356. doi:10.1016/j.gca.2018.06.029. ISSN 0016-7037. 
  6. ↑ Eiler, John M. (October 2007). ""Clumped-isotope" geochemistry—The study of naturally-occurring, multiply-substituted isotopologues". Earth and Planetary Science Letters 262 (3–4): 309–327. doi:10.1016/j.epsl.2007.08.020. ISSN 0012-821X. 
  7. ↑ 7.0 7.1 7.2 Cao, Xiaobin; Liu, Yun (January 2012). "Theoretical estimation of the equilibrium distribution of clumped isotopes in nature". Geochimica et Cosmochimica Acta 77: 292–303. doi:10.1016/j.gca.2011.11.021. ISSN 0016-7037. 
  8. ↑ 8.0 8.1 8.2 Piasecki, Alison; Sessions, Alex; Peterson, Brian; Eiler, John (October 2016). "Prediction of equilibrium distributions of isotopologues for methane, ethane and propane using density functional theory". Geochimica et Cosmochimica Acta 190: 1–12. doi:10.1016/j.gca.2016.06.003. ISSN 0016-7037. https://authors.library.caltech.edu/68833/2/mmc1%285%29.docx. 
  9. ↑ 9.0 9.1 9.2 9.3 9.4 9.5 9.6 9.7 9.8 9.9 Wang, David T.; Gruen, Danielle S.; Lollar, Barbara Sherwood; Hinrichs, Kai-Uwe; Stewart, Lucy C.; Holden, James F.; Hristov, Alexander N.; Pohlman, John W. et al. (2015-04-24). "Nonequilibrium clumped isotope signals in microbial methane" (in en). Science 348 (6233): 428–431. doi:10.1126/science.aaa4326. ISSN 0036-8075. PMID 25745067. 
  10. ↑ 10.0 10.1 10.2 10.3 10.4 10.5 10.6 10.7 Stolper, D. A.; Lawson, M.; Davis, C. L.; Ferreira, A. A.; Neto, E. V. Santos; Ellis, G. S.; Lewan, M. D.; Martini, A. M. et al. (2014-06-27). "Formation temperatures of thermogenic and biogenic methane" (in en). Science 344 (6191): 1500–1503. doi:10.1126/science.1254509. ISSN 0036-8075. PMID 24970083. https://authors.library.caltech.edu/46072/1/Stolper%20et%20al%2C%20accepted.pdf. 
  11. ↑ 11.0 11.1 Webb, Michael A.; Miller, Thomas F. (2014-01-03). "Position-Specific and Clumped Stable Isotope Studies: Comparison of the Urey and Path-Integral Approaches for Carbon Dioxide, Nitrous Oxide, Methane, and Propane" (in EN). The Journal of Physical Chemistry A 118 (2): 467–474. doi:10.1021/jp411134v. ISSN 1089-5639. PMID 24372450. https://authors.library.caltech.edu/43327/7/jp411134v_si_001.pdf. 
  12. ↑ 12.0 12.1 12.2 12.3 12.4 12.5 12.6 12.7 Young, E.D.; Kohl, I.E.; Lollar, B. Sherwood; Etiope, G.; Rumble, D.; Li (李姝宁), S.; Haghnegahdar, M.A.; Schauble, E.A. et al. (April 2017). "The relative abundances of resolved l2 CH 2 D 2 and 13 CH 3 D and mechanisms controlling isotopic bond ordering in abiotic and biotic methane gases". Geochimica et Cosmochimica Acta 203: 235–264. doi:10.1016/j.gca.2016.12.041. ISSN 0016-7037. 
  13. ↑ 13.0 13.1 13.2 13.3 13.4 13.5 13.6 13.7 Stolper, D.A.; Martini, A.M.; Clog, M.; Douglas, P.M.; Shusta, S.S.; Valentine, D.L.; Sessions, A.L.; Eiler, J.M. (July 2015). "Distinguishing and understanding thermogenic and biogenic sources of methane using multiply substituted isotopologues". Geochimica et Cosmochimica Acta 161: 219–247. doi:10.1016/j.gca.2015.04.015. ISSN 0016-7037. 
  14. ↑ 14.0 14.1 14.2 14.3 14.4 14.5 Douglas, P.M.J.; Stolper, D.A.; Smith, D.A.; Walter Anthony, K.M.; Paull, C.K.; Dallimore, S.; Wik, M.; Crill, P.M. et al. (September 2016). "Diverse origins of Arctic and Subarctic methane point source emissions identified with multiply-substituted isotopologues". Geochimica et Cosmochimica Acta 188: 163–188. doi:10.1016/j.gca.2016.05.031. ISSN 0016-7037. https://escholarship.org/uc/item/5t357148. 
  15. ↑ Eiler, John M.; Clog, Matthieu; Magyar, Paul; Piasecki, Alison; Sessions, Alex; Stolper, Daniel; Deerberg, Michael; Schlueter, Hans-Juergen et al. (February 2013). "A high-resolution gas-source isotope ratio mass spectrometer". International Journal of Mass Spectrometry 335: 45–56. doi:10.1016/j.ijms.2012.10.014. ISSN 1387-3806. 
  16. ↑ Young, Edward D.; Rumble, Douglas; Freedman, Philip; Mills, Mark (April 2016). "A large-radius high-mass-resolution multiple-collector isotope ratio mass spectrometer for analysis of rare isotopologues of O 2 , N 2 , CH 4 and other gases". International Journal of Mass Spectrometry 401: 1–10. doi:10.1016/j.ijms.2016.01.006. ISSN 1387-3806. 
  17. ↑ 17.0 17.1 Ono, Shuhei; Wang, David T.; Gruen, Danielle S.; Sherwood Lollar, Barbara; Zahniser, Mark S.; McManus, Barry J.; Nelson, David D. (2014-06-18). "Measurement of a Doubly Substituted Methane Isotopologue, 13CH3D, by Tunable Infrared Laser Direct Absorption Spectroscopy" (in EN). Analytical Chemistry 86 (13): 6487–6494. doi:10.1021/ac5010579. ISSN 0003-2700. PMID 24895840. http://dspace.mit.edu/bitstream/1721.1/98875/1/Ono%20-%20EAPS%20-%20Ono%20et%20al%202014.pdf. 
  18. ↑ Inagaki, F.; Hinrichs, K.-U.; Kubo, Y.; Bowles, M. W.; Heuer, V. B.; Hong, W.-L.; Hoshino, T.; Ijiri, A. et al. (2015-07-24). "Exploring deep microbial life in coal-bearing sediment down to ~2.5 km below the ocean floor" (in en). Science 349 (6246): 420–424. doi:10.1126/science.aaa6882. ISSN 0036-8075. PMID 26206933. 
  19. ↑ Shuai, Yanhua; Douglas, Peter M.J.; Zhang, Shuichang; Stolper, Daniel A.; Ellis, Geoffrey S.; Lawson, Michael; Lewan, Michael D.; Formolo, Michael et al. (February 2018). "Equilibrium and non-equilibrium controls on the abundances of clumped isotopologues of methane during thermogenic formation in laboratory experiments: Implications for the chemistry of pyrolysis and the origins of natural gases". Geochimica et Cosmochimica Acta 223: 159–174. doi:10.1016/j.gca.2017.11.024. ISSN 0016-7037. https://escholarship.org/uc/item/5qr487hm. 
  20. ↑ Wang, David T.; Reeves, Eoghan P.; McDermott, Jill M.; Seewald, Jeffrey S.; Ono, Shuhei (February 2018). "Clumped isotopologue constraints on the origin of methane at seafloor hot springs". Geochimica et Cosmochimica Acta 223: 141–158. doi:10.1016/j.gca.2017.11.030. ISSN 0016-7037. http://darchive.mblwhoilibrary.org/bitstream/1912/9625/1/WangEtAl_2018_GCA_forWHOAS_embd.pdf. 
  21. ↑ Shuai, Yanhua; Etiope, Giuseppe; Zhang, Shuichang; Douglas, Peter M.J.; Huang, Ling; Eiler, John M. (January 2018). "Methane clumped isotopes in the Songliao Basin (China): New insights into abiotic vs. biotic hydrocarbon formation". Earth and Planetary Science Letters 482: 213–221. doi:10.1016/j.epsl.2017.10.057. ISSN 0012-821X. https://authors.library.caltech.edu/84846/2/1-s2.0-S0012821X17306258-mmc1.doc. 
  22. ↑ Whiticar, Michael J. (1999-09-30). "Carbon and hydrogen isotope systematics of bacterial formation and oxidation of methane" (in en). Chemical Geology 161 (1–3): 291–314. doi:10.1016/S0009-2541(99)00092-3. ISSN 0009-2541. 



Retrieved from "https://handwiki.org/wiki/index.php?title=Physics:Methane_clumped_isotopes&oldid=3283262"