5-cell honeycomb
| 4-simplex honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Family | Simplectic honeycomb |
| Schläfli symbol | {3[5]} |
| Coxeter diagram | |
| 4-face types | {3,3,3} t1{3,3,3} |
| Cell types | {3,3} t1{3,3} |
| Face types | {3} |
| Vertex figure | 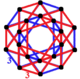 t0,3{3,3,3} |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
In four-dimensional Euclidean geometry, the 4-simplex honeycomb, 5-cell honeycomb or pentachoric-dispentachoric honeycomb is a space-filling tessellation honeycomb. It is composed of 5-cells and rectified 5-cells facets in a ratio of 1:1.
Structure
Cells of the vertex figure are ten tetrahedrons and 20 triangular prisms, corresponding to the ten 5-cells and 20 rectified 5-cells that meet at each vertex. All the vertices lie in parallel realms in which they form alternated cubic honeycombs, the tetrahedra being either tops of the rectified 5-cell or the bases of the 5-cell, and the octahedra being the bottoms of the rectified 5-cell.[1]
Alternate names
- Cyclopentachoric tetracomb
- Pentachoric-dispentachoric tetracomb
Projection by folding
The 5-cell honeycomb can be projected into the 2-dimensional square tiling by a geometric folding operation that maps two pairs of mirrors into each other, sharing the same vertex arrangement:
| [math]\displaystyle{ {\tilde{A}}_3 }[/math] | |
|---|---|
| [math]\displaystyle{ {\tilde{C}}_2 }[/math] |
A4 lattice
The vertex arrangement of the 5-cell honeycomb is called the A4 lattice, or 4-simplex lattice. The 20 vertices of its vertex figure, the runcinated 5-cell represent the 20 roots of the [math]\displaystyle{ {\tilde{A}}_4 }[/math] Coxeter group.[2][3] It is the 4-dimensional case of a simplectic honeycomb.
The A*4 lattice[4] is the union of five A4 lattices, and is the dual to the omnitruncated 5-simplex honeycomb, and therefore the Voronoi cell of this lattice is an omnitruncated 5-cell


 ∪
∪ 

 ∪
∪ 


 ∪
∪ 


 ∪
∪ 


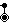 = dual of
= dual of 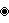

Related polytopes and honeycombs
The tops of the 5-cells in this honeycomb adjoin the bases of the 5-cells, and vice versa, in adjacent laminae (or layers); but alternating laminae may be inverted so that the tops of the rectified 5-cells adjoin the tops of the rectified 5-cells and the bases of the 5-cells adjoin the bases of other 5-cells. This inversion results in another non-Wythoffian uniform convex honeycomb. Octahedral prisms and tetrahedral prisms may be inserted in between alternated laminae as well, resulting in two more non-Wythoffian elongated uniform honeycombs.[5]
Rectified 5-cell honeycomb
| Rectified 5-cell honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Schläfli symbol | t0,2{3[5]} or r{3[5]} |
| Coxeter diagram | |
| 4-face types | t1{33} t0,2{33} 40px t0,3{33} |
| Cell types | Tetrahedron Octahedron 20px Cuboctahedron 20px Triangular prism |
| Vertex figure | triangular elongated-antiprismatic prism |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
The rectified 4-simplex honeycomb or rectified 5-cell honeycomb is a space-filling tessellation honeycomb.
Alternate names
- small cyclorhombated pentachoric tetracomb
- small prismatodispentachoric tetracomb
Cyclotruncated 5-cell honeycomb
| Cyclotruncated 5-cell honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Family | Truncated simplectic honeycomb |
| Schläfli symbol | t0,1{3[5]} |
| Coxeter diagram | |
| 4-face types | {3,3,3} t{3,3,3} 40px 2t{3,3,3} |
| Cell types | {3,3} t{3,3} |
| Face types | Triangle {3} Hexagon {6} |
| Vertex figure | 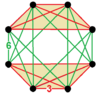 Tetrahedral antiprism [3,4,2+], order 48 |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
The cyclotruncated 4-simplex honeycomb or cyclotruncated 5-cell honeycomb is a space-filling tessellation honeycomb. It can also be seen as a birectified 5-cell honeycomb.
It is composed of 5-cells, truncated 5-cells, and bitruncated 5-cells facets in a ratio of 2:2:1. Its vertex figure is a tetrahedral antiprism, with 2 regular tetrahedron, 8 triangular pyramid, and 6 tetragonal disphenoid cells, defining 2 5-cell, 8 truncated 5-cell, and 6 bitruncated 5-cell facets around a vertex.
It can be constructed as five sets of parallel hyperplanes that divide space into two half-spaces. The 3-space hyperplanes contain quarter cubic honeycombs as a collection facets.[6]
Alternate names
- Cyclotruncated pentachoric tetracomb
- Small truncated-pentachoric tetracomb
Truncated 5-cell honeycomb
| Truncated 4-simplex honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Schläfli symbol | t0,1,2{3[5]} or t{3[5]} |
| Coxeter diagram | |
| 4-face types | t0,1{33} t0,1,2{33} 40px t0,3{33} |
| Cell types | Tetrahedron Truncated tetrahedron 20px Truncated octahedron 20px Triangular prism |
| Vertex figure | triangular elongated-antiprismatic pyramid |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
The truncated 4-simplex honeycomb or truncated 5-cell honeycomb is a space-filling tessellation honeycomb. It can also be called a cyclocantitruncated 5-cell honeycomb.
Alaternate names
- Great cyclorhombated pentachoric tetracomb
- Great truncated-pentachoric tetracomb
Cantellated 5-cell honeycomb
| Cantellated 5-cell honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Schläfli symbol | t0,1,3{3[5]} or rr{3[5]} |
| Coxeter diagram | |
| 4-face types | t0,2{33} t1,2{33} 40px t0,1,3{33} |
| Cell types | Truncated tetrahedron Octahedron 20px Cuboctahedron 20px Triangular prism 20px Hexagonal prism |
| Vertex figure | Bidiminished rectified pentachoron |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
The cantellated 4-simplex honeycomb or cantellated 5-cell honeycomb is a space-filling tessellation honeycomb. It can also be called a cycloruncitruncated 5-cell honeycomb.
Alternate names
- Cycloprismatorhombated pentachoric tetracomb
- Great prismatodispentachoric tetracomb
Bitruncated 5-cell honeycomb
| Bitruncated 5-cell honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Schläfli symbol | t0,1,2,3{3[5]} or 2t{3[5]} |
| Coxeter diagram | |
| 4-face types | t0,1,3{33} t0,1,2{33} 40px t0,1,2,3{33} |
| Cell types | Cuboctahedron Truncated octahedron |
| Vertex figure | tilted rectangular duopyramid |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×2, {3[5]} |
| Properties | vertex-transitive |
The bitruncated 4-simplex honeycomb or bitruncated 5-cell honeycomb is a space-filling tessellation honeycomb. It can also be called a cycloruncicantitruncated 5-cell honeycomb.
Alternate names
- Great cycloprismated pentachoric tetracomb
- Grand prismatodispentachoric tetracomb
Omnitruncated 5-cell honeycomb
| Omnitruncated 4-simplex honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform 4-honeycomb |
| Family | Omnitruncated simplectic honeycomb |
| Schläfli symbol | t0,1,2,3,4{3[5]} or tr{3[5]} |
| Coxeter diagram | |
| 4-face types | t0,1,2,3{3,3,3} |
| Cell types | t0,1,2{3,3} {6}x{} |
| Face types | {4} {6} |
| Vertex figure | 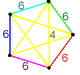 Irr. 5-cell |
| Symmetry | [math]\displaystyle{ {\tilde{A}}_4 }[/math]×10, [5[3[5]]] |
| Properties | vertex-transitive, cell-transitive |
The omnitruncated 4-simplex honeycomb or omnitruncated 5-cell honeycomb is a space-filling tessellation honeycomb. It can also be seen as a cyclosteriruncicantitruncated 5-cell honeycomb. .
It is composed entirely of omnitruncated 5-cell (omnitruncated 4-simplex) facets.
Coxeter calls this Hinton's honeycomb after C. H. Hinton, who described it in his book The Fourth Dimension in 1906.[7]
The facets of all omnitruncated simplectic honeycombs are called permutohedra and can be positioned in n+1 space with integral coordinates, permutations of the whole numbers (0,1,..,n).
Alternate names
- Omnitruncated cyclopentachoric tetracomb
- Great-prismatodecachoric tetracomb
A4* lattice
The A*4 lattice is the union of five A4 lattices, and is the dual to the omnitruncated 5-cell honeycomb, and therefore the Voronoi cell of this lattice is an omnitruncated 5-cell.[8]


 ∪
∪ 

 ∪
∪ 


 ∪
∪ 


 ∪
∪ 


 = dual of
= dual of 

Alternated form
This honeycomb can be alternated, creating omnisnub 5-cells with irregular 5-cells created at the deleted vertices. Although it is not uniform, the 5-cells have a symmetry of order 10.
See also
Regular and uniform honeycombs in 4-space:
- Tesseractic honeycomb
- 16-cell honeycomb
- 24-cell honeycomb
- Truncated 24-cell honeycomb
- Snub 24-cell honeycomb
Notes
- ↑ Olshevsky (2006), Model 134
- ↑ "The Lattice A4". http://www.math.rwth-aachen.de/~Gabriele.Nebe/LATTICES/A4.html.
- ↑ "A4 root lattice - Wolfram|Alpha". https://m.wolframalpha.com/input/?i=A4+root+lattice&lk=3.
- ↑ "The Lattice A4". http://www.math.rwth-aachen.de/~Gabriele.Nebe/LATTICES/As4.html.
- ↑ Olshevsky (2006), Klitzing, elong( x3o3o3o3o3*a ) - ecypit - O141, schmo( x3o3o3o3o3*a ) - zucypit - O142, elongschmo( x3o3o3o3o3*a ) - ezucypit - O143
- ↑ Olshevsky, (2006) Model 135
- ↑ The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. (The classification of Zonohededra, page 73)
- ↑ The Lattice A4*
References
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN:978-0-471-01003-6 [1]
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380–407, MR 2,10] (1.9 Uniform space-fillings)
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs) Model 134
- Klitzing, Richard. "4D Euclidean tesselations". https://bendwavy.org/klitzing/dimensions/flat.htm., x3o3o3o3o3*a - cypit - O134, x3x3x3x3x3*a - otcypit - 135, x3x3x3o3o3*a - gocyropit - O137, x3x3o3x3o3*a - cypropit - O138, x3x3x3x3o3*a - gocypapit - O139, x3x3x3x3x3*a - otcypit - 140
- Affine Coxeter group Wa(A4), Quaternions, and Decagonal Quasicrystals, Mehmet Koca, Nazife O. Koca, Ramazan Koc (2013) arXiv:1209.1878
Fundamental convex regular and uniform honeycombs in dimensions 2-9
| ||||||
|---|---|---|---|---|---|---|
| Space | Family | [math]\displaystyle{ {\tilde{A}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{C}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{B}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{D}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{G}}_2 }[/math] / [math]\displaystyle{ {\tilde{F}}_4 }[/math] / [math]\displaystyle{ {\tilde{E}}_{n-1} }[/math] |
| E2 | Uniform tiling | {3[3]} | δ3 | hδ3 | qδ3 | Hexagonal |
| E3 | Uniform convex honeycomb | {3[4]} | δ4 | hδ4 | qδ4 | |
| E4 | Uniform 4-honeycomb | {3[5]} | δ5 | hδ5 | qδ5 | 24-cell honeycomb |
| E5 | Uniform 5-honeycomb | {3[6]} | δ6 | hδ6 | qδ6 | |
| E6 | Uniform 6-honeycomb | {3[7]} | δ7 | hδ7 | qδ7 | 222 |
| E7 | Uniform 7-honeycomb | {3[8]} | δ8 | hδ8 | qδ8 | 133 • 331 |
| E8 | Uniform 8-honeycomb | {3[9]} | δ9 | hδ9 | qδ9 | 152 • 251 • 521 |
| E9 | Uniform 9-honeycomb | {3[10]} | δ10 | hδ10 | qδ10 | |
| En-1 | Uniform (n-1)-honeycomb | {3[n]} | δn | hδn | qδn | 1k2 • 2k1 • k21 |
 |

