Quarter hypercubic honeycomb
From HandWiki
In geometry, the quarter hypercubic honeycomb (or quarter n-cubic honeycomb) is a dimensional infinite series of honeycombs, based on the hypercube honeycomb. It is given a Schläfli symbol q{4,3...3,4} or Coxeter symbol qδ4 representing the regular form with three quarters of the vertices removed and containing the symmetry of Coxeter group [math]\displaystyle{ {\tilde{D}}_{n-1} }[/math] for n ≥ 5, with [math]\displaystyle{ {\tilde{D}}_4 }[/math] = [math]\displaystyle{ {\tilde{A}}_4 }[/math] and for quarter n-cubic honeycombs [math]\displaystyle{ {\tilde{D}}_5 }[/math] = [math]\displaystyle{ {\tilde{B}}_5 }[/math].[1]
| qδn | Name | Schläfli symbol |
Coxeter diagrams | Facets | Vertex figure | ||
|---|---|---|---|---|---|---|---|
| qδ3 |  quarter square tiling |
q{4,4} |
|
h{4}={2} | { }×{ } | { }×{ } | |
| qδ4 | 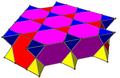 quarter cubic honeycomb |
q{4,3,4} |  h{4,3} |
60px h2{4,3} |
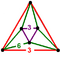 Elongated triangular antiprism | ||
| qδ5 | quarter tesseractic honeycomb | q{4,32,4} | 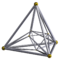 h{4,32} |
60px h3{4,32} |
{3,4}×{} | ||
| qδ6 | quarter 5-cubic honeycomb | q{4,33,4} |  h{4,33} |
 h4{4,33} |
 Rectified 5-cell antiprism | ||
| qδ7 | quarter 6-cubic honeycomb | q{4,34,4} |  h{4,34} |
 h5{4,34} |
{3,3}×{3,3} | ||
| qδ8 | quarter 7-cubic honeycomb | q{4,35,4} |  h{4,35} |
 h6{4,35} |
{3,3}×{3,31,1} | ||
| qδ9 | quarter 8-cubic honeycomb | q{4,36,4} |  h{4,36} |
 h7{4,36} |
{3,3}×{3,32,1} {3,31,1}×{3,31,1} | ||
| qδn | quarter n-cubic honeycomb | q{4,3n-3,4} | ... | h{4,3n-2} | hn-2{4,3n-2} | ... | |
See also
- Hypercubic honeycomb
- Alternated hypercubic honeycomb
- Simplectic honeycomb
- Truncated simplectic honeycomb
- Omnitruncated simplectic honeycomb
References
- ↑ Coxeter, Regular and semi-regular honeycoms, 1988, p.318-319
- Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
- pp. 122–123, 1973. (The lattice of hypercubes γn form the cubic honeycombs, δn+1)
- pp. 154–156: Partial truncation or alternation, represented by q prefix
- p. 296, Table II: Regular honeycombs, δn+1
- Kaleidoscopes: Selected Writings of H. S. M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 Uniform space-fillings)
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45] See p318 [2]
- Klitzing, Richard. "1D-8D Euclidean tesselations". https://bendwavy.org/klitzing/dimensions/flat.htm.
Fundamental convex regular and uniform honeycombs in dimensions 2-9
| ||||||
|---|---|---|---|---|---|---|
| Space | Family | [math]\displaystyle{ {\tilde{A}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{C}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{B}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{D}}_{n-1} }[/math] | [math]\displaystyle{ {\tilde{G}}_2 }[/math] / [math]\displaystyle{ {\tilde{F}}_4 }[/math] / [math]\displaystyle{ {\tilde{E}}_{n-1} }[/math] |
| E2 | Uniform tiling | {3[3]} | δ3 | hδ3 | qδ3 | Hexagonal |
| E3 | Uniform convex honeycomb | {3[4]} | δ4 | hδ4 | qδ4 | |
| E4 | Uniform 4-honeycomb | {3[5]} | δ5 | hδ5 | qδ5 | 24-cell honeycomb |
| E5 | Uniform 5-honeycomb | {3[6]} | δ6 | hδ6 | qδ6 | |
| E6 | Uniform 6-honeycomb | {3[7]} | δ7 | hδ7 | qδ7 | 222 |
| E7 | Uniform 7-honeycomb | {3[8]} | δ8 | hδ8 | qδ8 | 133 • 331 |
| E8 | Uniform 8-honeycomb | {3[9]} | δ9 | hδ9 | qδ9 | 152 • 251 • 521 |
| E9 | Uniform 9-honeycomb | {3[10]} | δ10 | hδ10 | qδ10 | |
| En-1 | Uniform (n-1)-honeycomb | {3[n]} | δn | hδn | qδn | 1k2 • 2k1 • k21 |
 |

