Physics:Coulomb constant

Short description: Proportionality constant in electrodynamics equations

Value of k	Units
8.9875517923(14)×109	N·m2/C2
14.3996	eV·Å·e−2
10−7	(N·s2/C2)c2

The Coulomb constant, the electric force constant, or the electrostatic constant (denoted k_e, k or K) is a proportionality constant in electrostatics equations. In SI base units it is equal to 8.9875517923(14)×10⁹ kg⋅m³⋅s⁻⁴⋅A⁻². It was named after the French physicist Charles-Augustin de Coulomb (1736–1806) who introduced Coulomb's law.

Value of the constant

The Coulomb constant is the constant of proportionality in Coulomb's law,

[math]\displaystyle{ \mathbf{F} = k_\text{e}\frac{Qq}{r^2}\mathbf{\hat{e}}_r }[/math]

where ê_r is a unit vector in the r-direction. In SI:

[math]\displaystyle{ k_\text{e} = \frac{1}{4\pi\varepsilon_0}, }[/math]

where [math]\displaystyle{ \varepsilon_0 }[/math] is the vacuum permittivity. This formula can be derived from Gauss' law,

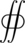[math]\displaystyle{ {\scriptstyle S} }[/math] [math]\displaystyle{ \mathbf{E} \cdot {\rm d}\mathbf{A} = \frac{Q}{\varepsilon_0} }[/math]

Taking this integral for a sphere, radius r, centered on a point charge, the electric field points radially outwards and is normal to a differential surface element on the sphere with constant magnitude for all points on the sphere.

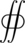[math]\displaystyle{ {\scriptstyle S} }[/math] [math]\displaystyle{ \mathbf{E} \cdot {\rm d}\mathbf{A} = |\mathbf{E}|\int_{S} dA = |\mathbf{E}| \times 4\pi r^{2} }[/math]

Noting that E = F/q for some test charge q,

[math]\displaystyle{ \begin{align} \mathbf{F} &= \frac{1}{4\pi\varepsilon_0}\frac{Qq}{r^2}\mathbf{\hat{e}}_r = k_\text{e}\frac{Qq}{r^2}\mathbf{\hat{e}}_r \\[8pt] \therefore k_\text{e} &= \frac{1}{4\pi\varepsilon_0} \end{align} }[/math]

Coulomb's law is an inverse-square law, and thereby similar to many other scientific laws ranging from gravitational pull to light attenuation. This law states that a specified physical quantity is inversely proportional to the square of the distance.[math]\displaystyle{ \text{intensity} = \frac{1}{d^2} }[/math]In some modern systems of units, the Coulomb constant k_e has an exact numeric value; in Gaussian units k_e = 1, in Heaviside–Lorentz units (also called rationalized) k_e = 1/4π. This was previously true in SI when the vacuum permeability was defined as μ₀ = 4π×10⁻⁷ H⋅m⁻¹. Together with the speed of light in vacuum c, defined as 299792458 m/s, the vacuum permittivity ε₀ can be written as 1/μ₀c², which gave an exact value of

[math]\displaystyle{ \begin{align} k_\text{e} = \frac{1}{4\pi\varepsilon_0}=\frac{c^2\mu_0}{4\pi}&=c^2\times (10^{-7}\ \mathrm{H{\cdot}m}^{-1})\\ &= 8.987\ 551\ 787\ 368\ 1764\times 10^9~\mathrm{N{\cdot}m^2{\cdot}C^{-2}}. \end{align} }[/math]

Since the redefinition of SI base units, the Coulomb constant is no longer exactly defined and is subject to the measurement error in the fine structure constant, as calculated from CODATA 2018 recommended values being

[math]\displaystyle{ k_\text{e} = 8.987\,551\,7923\,(14)\times 10^9\;\mathrm{kg{\cdot}m^{3}{\cdot}s^{-4}{\cdot}A^{-2}} . }[/math]

Use

The Coulomb constant is used in many electric equations, although it is sometimes expressed as the following product of the vacuum permittivity constant:

[math]\displaystyle{ k_\text{e} = \frac{1}{4 \pi \varepsilon_0}. }[/math]

The Coulomb constant appears in many expressions including the following:

Coulomb's law

[math]\displaystyle{ \mathbf{F}=k_\text{e}{Qq\over r^2}\mathbf{\hat{e}}_r. }[/math]

Electric potential energy

[math]\displaystyle{ U_\text{E}(r) = k_\text{e}\frac{Qq}{r}. }[/math]

Electric field

[math]\displaystyle{ \mathbf{E} = k_\text{e} \sum_{i=1}^N \frac{Q_i}{r_i^2} \mathbf{\hat{r}}_i. }[/math]

See also

• Gravitational constant
• Vacuum permittivity
• Vacuum permeability
• Inverse-square law