Physics:Lambda point

From HandWiki
Revision as of 19:20, 25 June 2023 by LinuxGuru (talk | contribs) (over-write)
(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)
The plot of the specific heat capacity versus temperature.

The lambda point is the temperature at which normal fluid helium (helium I) makes the transition to superfluid helium II (approximately 2.17 K at 1 atmosphere). The lowest pressure at which He-I and He-II can coexist is the vapor−He-I−He-II triple point at 2.1768 K (−270.9732 °C) and 5.0418 kPa (0.049759 atm), which is the "saturated vapor pressure" at that temperature (pure helium gas in thermal equilibrium over the liquid surface, in a hermetic container).[1] The highest pressure at which He-I and He-II can coexist is the bcc−He-I−He-II triple point with a helium solid at 1.762 K (−271.388 °C), 29.725 atm (3,011.9 kPa).[2]

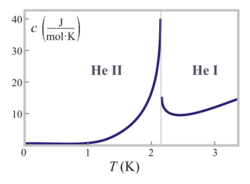
The point's name derives from the graph (pictured) that results from plotting the specific heat capacity as a function of temperature (for a given pressure in the above range, in the example shown, at 1 atmosphere), which resembles the Greek letter lambda [math]\displaystyle{ \lambda }[/math]. The specific heat capacity has a sharp peak as the temperature approaches the lambda point. The tip of the peak is so sharp that a critical exponent characterizing the divergence of the heat capacity can be measured precisely only in zero gravity, to provide a uniform density over a substantial volume of fluid. Hence the heat capacity was measured within 2 nK below the transition in an experiment included in a Space Shuttle payload in 1992.[3]

Question, Web Fundamentals.svg Unsolved problem in physics:
Explain the discrepancy between the experimental and theoretical determinations of the heat capacity critical exponent α for the superfluid transition in helium-4.[4]
(more unsolved problems in physics)

Although the heat capacity has a peak, it does not tend towards infinity (contrary to what the graph may suggest), but has finite limiting values when approaching the transition from above and below.[3] The behavior of the heat capacity near the peak is described by the formula [math]\displaystyle{ C\approx A_\pm t^{-\alpha}+B_\pm }[/math] where [math]\displaystyle{ t=|1-T/T_c| }[/math] is the reduced temperature, [math]\displaystyle{ T_c }[/math] is the Lambda point temperature, [math]\displaystyle{ A_\pm,B_\pm }[/math] are constants (different above and below the transition temperature), and α is the critical exponent: [math]\displaystyle{ \alpha=-0.0127(3) }[/math].[3][5] Since this exponent is negative for the superfluid transition, specific heat remains finite.[6]

The quoted experimental value of α is in a significant disagreement[7][4] with the most precise theoretical determinations[8][9][10] coming from high temperature expansion techniques, Monte Carlo methods and the conformal bootstrap.

See also

References

  1. ↑ Donnelly, Russell J.; Barenghi, Carlo F. (1998). "The Observed Properties of Liquid Helium at the Saturated Vapor Pressure". Journal of Physical and Chemical Reference Data 27 (6): 1217–1274. doi:10.1063/1.556028. Bibcode: 1998JPCRD..27.1217D. 
  2. ↑ Hoffer, J. K.; Gardner, W. R.; Waterfield, C. G.; Phillips, N. E. (April 1976). "Thermodynamic properties of 4He. II. The bcc phase and the P-T and VT phase diagrams below 2 K". Journal of Low Temperature Physics 23 (1): 63–102. doi:10.1007/BF00117245. Bibcode: 1976JLTP...23...63H. 
  3. ↑ 3.0 3.1 3.2 Lipa, J.A.; Swanson, D. R.; Nissen, J. A.; Chui, T. C. P.; Israelsson, U. E. (1996). "Heat Capacity and Thermal Relaxation of Bulk Helium very near the Lambda Point". Physical Review Letters 76 (6): 944–7. doi:10.1103/PhysRevLett.76.944. PMID 10061591. Bibcode: 1996PhRvL..76..944L. 
  4. ↑ 4.0 4.1 Rychkov, Slava (2020-01-31). "Conformal bootstrap and the λ-point specific heat experimental anomaly" (in en). Journal Club for Condensed Matter Physics. doi:10.36471/JCCM_January_2020_02. https://www.condmatjclub.org/?p=4037. 
  5. ↑ Lipa, J. A.; Nissen, J. A.; Stricker, D. A.; Swanson, D. R.; Chui, T. C. P. (2003-11-14). "Specific heat of liquid helium in zero gravity very near the lambda point". Physical Review B 68 (17): 174518. doi:10.1103/PhysRevB.68.174518. Bibcode: 2003PhRvB..68q4518L. 
  6. ↑ For other phase transitions [math]\displaystyle{ \alpha }[/math] may be negative (e.g. [math]\displaystyle{ \alpha\approx+0.1 }[/math] for the liquid-vapor critical point which has Ising critical exponents). For those phase transitions specific heat does tend to infinity.
  7. ↑ Vicari, Ettore (2008-03-21). "Critical phenomena and renormalization-group flow of multi-parameter Phi4 theories" (in en). Proceedings of the XXV International Symposium on Lattice Field Theory — PoS(LATTICE 2007) (Regensburg, Germany: Sissa Medialab) 42: 023. doi:10.22323/1.042.0023. 
  8. ↑ Campostrini, Massimo; Hasenbusch, Martin; Pelissetto, Andrea; Vicari, Ettore (2006-10-06). "Theoretical estimates of the critical exponents of the superfluid transition in $^{4}\mathrm{He}$ by lattice methods". Physical Review B 74 (14): 144506. doi:10.1103/PhysRevB.74.144506. 
  9. ↑ Hasenbusch, Martin (2019-12-26). "Monte Carlo study of an improved clock model in three dimensions". Physical Review B 100 (22): 224517. doi:10.1103/PhysRevB.100.224517. ISSN 2469-9950. Bibcode: 2019PhRvB.100v4517H. 
  10. ↑ Chester, Shai M.; Landry, Walter; Liu, Junyu; Poland, David; Simmons-Duffin, David; Su, Ning; Vichi, Alessandro (2020). "Carving out OPE space and precise O(2) model critical exponents". Journal of High Energy Physics 2020 (6): 142. doi:10.1007/JHEP06(2020)142. Bibcode: 2020JHEP...06..142C. 

External links



Retrieved from "https://handwiki.org/wiki/index.php?title=Physics:Lambda_point&oldid=2935663"