Kelvin
| kelvin | |
|---|---|
 Thermometer with markings in degrees Celsius and in kelvins | |
| General information | |
| Unit system | SI |
| Unit of | temperature |
| Symbol | K |
| Named after | William Thomson, 1st Baron Kelvin |
| Conversions | |
| x K in ... | ... corresponds to ... |
| Celsius | (x − 273.15) °C |
| Fahrenheit | (1.8 x − 459.67) °F |
| Rankine | 1.8 x °Ra |
The kelvin, symbol K, is a unit of measurement for temperature.[1] The Kelvin scale is an absolute scale, which is defined such that 0 K is absolute zero and a change of thermodynamic temperature T by 1 kelvin corresponds to a change of thermal energy kT by 1.380649×10−23 J. The Boltzmann constant k = 1.380649×10−23 J⋅K−1 was exactly defined in the 2019 redefinition of the SI base units such that the triple point of water is 273.16±0.0001 K.[2] The kelvin is the base unit of temperature in the International System of Units (SI), used alongside its prefixed forms.[2][3][4] It is named after the Belfast-born and University of Glasgow-based engineer and physicist William Thomson, 1st Baron Kelvin (1824–1907).[5]
Historically, the Kelvin scale was developed from the Celsius scale, such that 273.15 K was 0 °C (the approximate melting point of ice) and a change of one kelvin was exactly equal to a change of one degree Celsius.[1][5] This relationship remains accurate, but the Celsius, Fahrenheit, and Rankine scales are now defined in terms of the Kelvin scale.[2][6][7] The kelvin is the primary unit of temperature for engineering and the physical sciences, while in most countries the Celsius scale remains the dominant scale outside of these fields.[5] In the United States, outside of the physical sciences, the Fahrenheit scale predominates, with the kelvin or Rankine scale employed for absolute temperature.[6]
History
Precursors

During the 18th century, multiple temperature scales were developed,[8] notably Fahrenheit and centigrade (later Celsius). These scales predated much of the modern science of thermodynamics, including atomic theory and the kinetic theory of gases which underpin the concept of absolute zero. Instead, they chose defining points within the range of human experience that could be reproduced easily and with reasonable accuracy, but lacked any deep significance in thermal physics. In the case of the Celsius scale (and the long since defunct Newton scale and Réaumur scale) the melting point of water served as such a starting point, with Celsius being defined, from the 1740s up until the 1940s, by calibrating a thermometer such that
- The freezing point of water is 0 degrees.
- The boiling point of water is 100 degrees.
This definition assumes pure water at a specific pressure chosen to approximate the natural air pressure at sea level. Thus an increment of 1 °C equals 1/100 of the temperature difference between the melting and boiling points. The same temperature interval was later used for the Kelvin scale.
Charles's law
From 1787 to 1802, it was determined by Jacques Charles (unpublished), John Dalton,[9][10] and Joseph Louis Gay-Lussac[11] that, at constant pressure, ideal gases expanded or contracted their volume linearly (Charles's law) by about 1/273 parts per degree Celsius of temperature's change up or down, between 0 °C and 100 °C. This suggested that the volume of a gas cooled at about −273 °C would reach zero.
Lord Kelvin

In 1848, William Thomson, who was later ennobled as Lord Kelvin, published a paper On an Absolute Thermometric Scale.[12][13][14] Using the soon-to-be-defunct caloric theory, he proposed an "absolute" scale based on the following parameters:
- The melting point of water is 0 degrees.
- The boiling point of water is 100 degrees.
"The arbitrary points which coincide on the two scales are 0° and 100°"
- Any two heat engines whose heat source and heat sink are both separated by the same number of degrees will, per Carnot's theorem, be capable of producing the same amount of mechanical work per unit of "caloric" passing through.
"The characteristic property of the scale which I now propose is, that all degrees have the same value; that is, that a unit of heat descending from a body A at the temperature T° of this scale, to a body B at the temperature (T − 1)°, would give out the same mechanical effect, whatever be the number T. This may justly be termed an absolute scale, since its characteristic is quite independent of the physical properties of any specific substance."
As Carnot's theorem is understood in modern thermodynamics to simply describe the maximum efficiency with which thermal energy can be converted to mechanical energy and the predicted maximum efficiency is a function of the ratio between the absolute temperatures of the heat source and heat sink:
- Efficiency ≤ 1 − absolute temperate of heat sink/absolute temperature of heat source
It follows that increments of equal numbers of degrees on this scale must always represent equal proportional increases in absolute temperature. The numerical value of an absolute temperature, T, on the 1848 scale is related to the absolute temperature of the melting point of water, Tmpw, and the absolute temperature of the boiling point of water, Tbpw, by
- T (1848 scale) = 100 (ln T/Tmpw) / (ln Tbpw/Tmpw)
On this scale, an increase of 222 degrees always means an approximate doubling of absolute temperature regardless of the starting temperature.
In a footnote Thomson calculated that "infinite cold" (absolute zero, which would have a numerical value of negative infinity on this scale) was equivalent to −273 °C using the air thermometers of the time. This value of "−273" was the negative reciprocal of 0.00366—the accepted coefficient of thermal expansion of an ideal gas per degree Celsius relative to the ice point, giving a remarkable consistency to the currently accepted value.[15]
Within a decade, Thomson had abandoned caloric theory and superseded the 1848 scale with a new one[13][16] based on the 2 features that would characterise all future versions of the Kelvin scale:
- Absolute zero is the null point.
- Increments have the same magnitude as they do in the Celsius scale.
In 1892, Thomson was awarded the noble title 1st Baron Kelvin of Largs, or more succinctly Lord Kelvin. This name was a reference to the River Kelvin which flows through the grounds of Glasgow University.
In the early decades of the 20th century, the Kelvin scale was often called the "absolute Celsius" scale, indicating Celsius degrees counted from absolute zero rather than the freezing point of water, and using the same symbol for regular Celsius degrees, °C.[lower-alpha 1]
Triple point standard
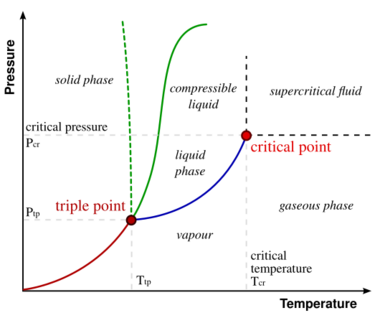
In 1873, William Thomson's older brother James coined the term triple point[17] to describe the combination of temperature and pressure at which the solid, liquid, and gas phases of a substance were capable of coexisting in thermodynamic equilibrium. While any two phases could coexist along a range of temperature-pressure combinations (e.g. the boiling point of water can be affected quite dramatically by raising or lowering the pressure), the triple point condition for a given substance can occur only at a single pressure and only at a single temperature. By the 1940s, the triple point of water had been experimentally measured to be about 0.6% of standard atmospheric pressure and very close to 0.01 °C per the historical definition of Celsius then in use.
In 1948, the Celsius scale was recalibrated by assigning the triple point temperature of water the value of 0.01 °C exactly[18] and allowing the melting point at standard atmospheric pressure to have an empirically determined value (and the actual melting point at ambient pressure to have a fluctuating value) close to 0 °C. This was justified on the grounds that the triple point was judged to give a more accurately reproducible reference temperature than the melting point.[19] The triple point could be measured with ±0.0001 °C accuracy, while the melting point just to ±0.001 °C.[18]
In 1954, with absolute zero having been experimentally determined to be about −273.15 °C per the definition of °C then in use, Resolution 3 of the 10th General Conference on Weights and Measures (CGPM) introduced a new internationally standardised Kelvin scale which defined the triple point as exactly 273.15 + 0.01 = 273.16 degrees Kelvin.[20][21]
In 1967/1968, Resolution 3 of the 13th CGPM renamed the unit increment of thermodynamic temperature "kelvin", symbol K, replacing "degree Kelvin", symbol °K.[22][23][24] The 13th CGPM also held in Resolution 4 that "The kelvin, unit of thermodynamic temperature, is equal to the fraction 1/273.16 of the thermodynamic temperature of the triple point of water."[4][25][26]
After the 1983 redefinition of the metre, this left the kelvin, the second, and the kilogram as the only SI units not defined with reference to any other unit.
In 2005, noting that the triple point could be influenced by the isotopic ratio of the hydrogen and oxygen making up a water sample and that this was "now one of the major sources of the observed variability between different realizations of the water triple point", the International Committee for Weights and Measures (CIPM), a committee of the CGPM, affirmed that for the purposes of delineating the temperature of the triple point of water, the definition of the kelvin would refer to water having the isotopic composition specified for Vienna Standard Mean Ocean Water.[4][27][28]
2019 redefinition
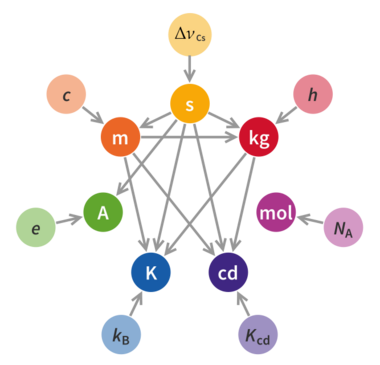
In 2005, the CIPM began a programme to redefine the kelvin (along with the other SI units) using a more experimentally rigorous method. In particular, the committee proposed redefining the kelvin such that the Boltzmann constant takes the exact value 1.3806505×10−23 J/K.[29] The committee had hoped that the program would be completed in time for its adoption by the CGPM at its 2011 meeting, but at the 2011 meeting the decision was postponed to the 2014 meeting when it would be considered as part of a larger program.[30]
The redefinition was further postponed in 2014, pending more accurate measurements of the Boltzmann constant in terms of the current definition,[31] but was finally adopted at the 26th CGPM in late 2018, with a value of k = 1.380649×10−23 J⋅K−1.[32][29][1][2][4][33]
For scientific purposes, the main advantage is that this allows measurements at very low and very high temperatures to be made more accurately, as the techniques used depend on the Boltzmann constant. It also has the philosophical advantage of being independent of any particular substance. The unit J/K is equal to kg⋅m2⋅s−2⋅K−1, where the kilogram, metre and second are defined in terms of the Planck constant, the speed of light, and the duration of the caesium-133 ground-state hyperfine transition respectively.[2] Thus, this definition depends only on universal constants, and not on any physical artifacts as practiced previously. The challenge was to avoid degrading the accuracy of measurements close to the triple point. For practical purposes, the redefinition was unnoticed; water still freezes at 273.15 K (0 °C),[2][34] and the triple point of water continues to be a commonly used laboratory reference temperature.
The difference is that, before the redefinition, the triple point of water was exact and the Boltzmann constant had a measured value of 1.38064903(51)×10−23 J/K, with a relative standard uncertainty of 3.7×10−7.[35] Afterward, the Boltzmann constant is exact and the uncertainty is transferred to the triple point of water, which is now 273.1600(1) K.
The new definition officially came into force on 20 May 2019, the 144th anniversary of the Metre Convention.[33][1][2][4]
Practical uses
Colour temperature
The kelvin is often used as a measure of the colour temperature of light sources. Colour temperature is based upon the principle that a black body radiator emits light with a frequency distribution characteristic of its temperature. Black bodies at temperatures below about 4000 K appear reddish, whereas those above about 7500 K appear bluish. Colour temperature is important in the fields of image projection and photography, where a colour temperature of approximately 5600 K is required to match "daylight" film emulsions. In astronomy, the stellar classification of stars and their place on the Hertzsprung–Russell diagram are based, in part, upon their surface temperature, known as effective temperature. The photosphere of the Sun, for instance, has an effective temperature of 5772 K [1][2][3][4] as adopted by IAU 2015 Resolution B3.
Digital cameras and photographic software often use colour temperature in K in edit and setup menus. The simple guide is that higher colour temperature produces an image with enhanced white and blue hues. The reduction in colour temperature produces an image more dominated by reddish, "warmer" colours.
Kelvin as a unit of noise temperature
For electronics, the kelvin is used as an indicator of how noisy a circuit is in relation to an ultimate noise floor, i.e. the noise temperature. The so-called Johnson–Nyquist noise of discrete resistors and capacitors is a type of thermal noise derived from the Boltzmann constant and can be used to determine the noise temperature of a circuit using the Friis formulas for noise.
Derived units and SI multiples
The only SI derived unit with a special name derived from the kelvin is the degree Celsius. Like other SI units, the kelvin can also be modified by adding a metric prefix that multiplies it by a power of 10:
| Submultiples | Multiples | |||||
|---|---|---|---|---|---|---|
| Value | SI symbol | Name | Value | SI symbol | Name | |
| 10−1 K | dK | decikelvin | 101 K | daK | decakelvin | |
| 10−2 K | cK | centikelvin | 102 K | hK | hectokelvin | |
| 10−3 K | mK | millikelvin | 103 K | kK | kilokelvin | |
| 10−6 K | µK | microkelvin | 106 K | MK | megakelvin | |
| 10−9 K | nK | nanokelvin | 109 K | GK | gigakelvin | |
| 10−12 K | pK | picokelvin | 1012 K | TK | terakelvin | |
| 10−15 K | fK | femtokelvin | 1015 K | PK | petakelvin | |
| 10−18 K | aK | attokelvin | 1018 K | EK | exakelvin | |
| 10−21 K | zK | zeptokelvin | 1021 K | ZK | zettakelvin | |
| 10−24 K | yK | yoctokelvin | 1024 K | YK | yottakelvin | |
Orthography
According to SI convention, the kelvin is never referred to nor written as a degree. The word "kelvin" is not capitalised when used as a unit. It is pluralised as appropriate (for example, "it is 283 kelvins outside", in contrast with "it is 50 degrees Fahrenheit" or "10 degrees Celsius").[36][37][38] The unit symbol K is a capital letter.[22] It is common convention to capitalize Kelvin when referring to Lord Kelvin[5] or the Kelvin scale.[39]
The unit symbol K is encoded in Unicode at code point U+212A K KELVIN SIGN. However, this is a compatibility character provided for compatibility with legacy encodings. The Unicode standard recommends using U+004B K LATIN CAPITAL LETTER K instead; that is, a normal capital K. "Three letterlike symbols have been given canonical equivalence to regular letters: U+2126 Ω OHM SIGN, U+212A K KELVIN SIGN, and U+212B Å ANGSTROM SIGN. In all three instances, the regular letter should be used."[40]
See also
- Outline of metrology and measurement
- Comparison of temperature scales
- International Temperature Scale of 1990
- Negative temperature
Notes
- ↑ For example, Encyclopaedia Britannica editions from the 1920s and 1950s, one example being the article "Planets".
References
- ↑ 1.0 1.1 1.2 1.3 BIPM (20 May 2019). "Mise en pratique for the definition of the kelvin in the SI". https://www.bipm.org/documents/20126/41489682/SI-App2-kelvin.pdf/cd36cb68-3f00-05fd-339e-452df0b6215e?version=1.5&t=1637237805352&download=false.
- ↑ 2.0 2.1 2.2 2.3 2.4 2.5 2.6 "SI Brochure: The International System of Units (SI) – 9th edition (updated in 2022)". BIPM. https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-EN.pdf/2d2b50bf-f2b4-9661-f402-5f9d66e4b507.
- ↑ "SI base unit: kelvin (K)". BIPM. https://www.bipm.org/en/si-base-units/kelvin.
- ↑ 4.0 4.1 4.2 4.3 4.4 "A Turning Point for Humanity: Redefining the World's Measurement System". NIST. 12 May 2018. https://www.nist.gov/si-redefinition/turning-point-humanity-redefining-worlds-measurement-system. Retrieved 21 February 2022.
- ↑ 5.0 5.1 5.2 5.3 "Kelvin: Introduction" (in en). 14 May 2018. https://www.nist.gov/si-redefinition/kelvin-introduction.
- ↑ 6.0 6.1 "Busting Myths about the Metric System". NIST (Taking Measure (official blog of the NIST)). 6 October 2020. https://www.nist.gov/blogs/taking-measure/busting-myths-about-metric-system. Retrieved 21 February 2022.
- ↑ "Handbook 44 – 2022 – Appendix C – General Tables of Units of Measurement". NIST. https://www.nist.gov/system/files/documents/2021/11/30/2022-HB44-Section-Appendix-C.pdf.
- ↑ "Kelvin: History". NIST. 14 May 2018. https://www.nist.gov/si-redefinition/kelvin-history. Retrieved 21 February 2022.
- ↑ "Essay II. On the force of steam or vapour from water and various other liquids, both in vacuum and in air". Memoirs of the Literary and Philosophical Society of Manchester 5 part 2: 550–574. 1801. https://books.google.com/books?id=3qdJAAAAYAAJ&pg=PA595.
- ↑ "Essay IV. On the expansion of elastic fluids by heat". Memoirs of the Literary and Philosophical Society of Manchester 5 part 2: 595–602. 1801. https://books.google.com/books?id=3qdJAAAAYAAJ&pg=PA595.
- ↑ "Recherches sur la dilatation des gaz et des vapeurs", Annales de Chimie XLIII: 137, 1802. English translation (extract).
- ↑ "On an Absolute Thermometric Scale founded on Carnot's Theory of the Motive Power of Heat, and calculated from Regnault's Observations". Philosophical Magazine. https://zapatopi.net/kelvin/papers/on_an_absolute_thermometric_scale.html.
- ↑ 13.0 13.1 "On an Absolute Thermometric Scale founded on Carnot's Theory of the Motive Power of Heat, and calculated from Regnault's Observations (1881 reprint)". Philosophical Magazine. https://www3.nd.edu/~powers/ame.20231/kelvin1848.pdf.
- ↑ "On an Absolute Thermometric Scale". Philosophical Magazine. October 1848. http://zapatopi.net/kelvin/papers/on_an_absolute_thermometric_scale.html. Retrieved 2008-02-06.
- ↑ "On an Absolute Thermometric Scale founded on Carnot's Theory of the Motive Power of Heat, and calculated from Regnault's Observations (1881 reprint)". Philosophical Magazine. https://www3.nd.edu/~powers/ame.20231/kelvin1848.pdf. "If we push the strict principle of graduation, stated above, sufficiently far, we should arrive at a point corresponding to the volume of air being reduced to nothing, which would be marked as -273° of the scale (-100/·366, if ·366 be the coefficient of expansion); and therefore -273° of the air-thermometer is a point which cannot be reached at any finite temperature, however low"
- ↑ Thomson, William. "On the Dynamical Theory of Heat, with numerical results deduced from Mr Joule's equivalent of a Thermal Unit, and M. Regnault's Observations on Steam (Excerpts)". Transactions of the Royal Society of Edinburgh and Philosophical Magazine. https://zapatopi.net/kelvin/papers/on_the_dynamical_theory_of_heat.html.
- ↑ Thomson, James (1873). "A quantitative investigation of certain relations between the gaseous, the liquid, and the solid states of water-substance". Proceedings of the Royal Society of London 22: 28. ISSN 0370-1662. Bibcode: 1873RSPS...22...27T. https://babel.hathitrust.org/cgi/pt?id=hvd.32044106377062&view=1up&seq=48. "and consequently that the three curves would meet or cross each other in one point, which I have called the triple point.".
- ↑ 18.0 18.1 Swinton, F. L. (September 1967). "The triplet point of water" (in en). Journal of Chemical Education 44 (9): 541. doi:10.1021/ed044p541. ISSN 0021-9584. Bibcode: 1967JChEd..44..541S. https://pubs.acs.org/doi/abs/10.1021/ed044p541.
- ↑ "Resolution 3 of the 9th CGPM (1948)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/9-1948/resolution-3.
- ↑ "Resolution 3 of the 10th CGPM (1954)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/10-1954/resolution-3.
- ↑ "Resolution 3: Definition of the thermodynamic temperature scale". Resolutions of the 10th CGPM. Bureau International des Poids et Mesures. 1954. http://www.bipm.fr/en/CGPM/db/10/3/.
- ↑ 22.0 22.1 "Resolution 3 of the 13th CGPM (1967)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/13-1967/resolution-3.
- ↑ "Resolution 3: SI unit of thermodynamic temperature (kelvin)". Resolutions of the 13th CGPM. Bureau International des Poids et Mesures. 1967. http://www.bipm.fr/en/CGPM/db/13/3/.
- ↑ "Nox, Dunkelleuchtdichte, Skot" (in de). Physikalisches Wörterbuch (1 ed.). Berlin / Göttingen / Heidelberg, Germany: Springer-Verlag OHG. 1952. pp. 125, 271, 389. doi:10.1007/978-3-662-12706-3. ISBN 978-3-662-12707-0. https://books.google.com/books?id=QaCFBwAAQBAJ&pg=RA1-PA125. Retrieved 2023-03-16. "Dunkelleuchtdichte. […] Unter Zugrundelegung dieser Empfindlichkeitskurve hat man 1940 in Deutschland die Dunkelleuchtdichte mit der Einheit Skot (sk) so festgesetzt, daß bei einem Licht der Farbtemperatur 2360 °K 1 sk = 10−3 asb gilt. 1948 ist von der Internationalen Beleuchtungskommission (IBK) die Bezugstemperatur auf 2046 °K, die Erstarrungstemperatur des Platins, festgesetzt worden. Die Bezeichnung Skot wurde von der IBK nicht übernommen, dafür soll "skotopisches Stilb" gesagt werden. Als höchstzulässiger Grenzwert für die Dunkelleuchtdichte ist in Deutschland 10 Skot festgesetzt worden, um eine Verwendung der Dunkelleuchtdichte im Gebiet des gemischten Zapfen- und Stäbchensehens zu vermeiden, da in diesem Bereich die photometrischen Maßgrößen wegen der allmählich gleitenden Augenempfindlichkeitskurve ihren Sinn verlieren. […] Skot, abgek[ürzt] sk, Einheit für die Dunkelleuchtdichte, welche für zahlenmäßige Angaben und zum Anschluß der Dunkelleuchtdichte an die normale Leuchtdichte 1940 von der German Lighting Society (de) geschaffen wurde. Für diesen Anschluß wurde die Strahlung des schwarzen Körpers bei T = 2360 °K, d.h. eine Strahlung der Farbtemperatur T1 = 2360 °K vereinbart. Eine Lichtquelle strahlt mit der Dunkelleuchtdichte 1 sk, wenn sie photometrisch gleich einer Strahlung der Farbtemperatur T2 = 2360 °K und der Leuchtdichte von 10−3 asb (Apostilb) ist. Bei der Farbtemperatur T1 = 2360 °K gilt also die Relation: 1 sk = 10−3 asb = 10−7/π sb."
- ↑ "Resolution 4 of the 13th CGPM (1967)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/13-1967/resolution-4.
- ↑ "Resolution 4: Definition of the SI unit of thermodynamic temperature (kelvin)". Resolutions of the 13th CGPM. Bureau International des Poids et Mesures. 1967. https://www.bipm.org/en/CGPM/db/13/4/.
- ↑ "Resolution 10 of the 23rd CGPM (2007)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/23-2007/resolution-10.
- ↑ "Unit of thermodynamic temperature (kelvin)". SI Brochure, 8th edition. Bureau International des Poids et Mesures. 1967. pp. Section 2.1.1.5. http://www1.bipm.org/en/si/si_brochure/chapter2/2-1/2-1-1/kelvin.html.
- ↑ 29.0 29.1 Ian Mills (29 September 2010). "Draft Chapter 2 for SI Brochure, following redefinitions of the base units". CCU. http://www.bipm.org/utils/en/pdf/si_brochure_draft_ch2.pdf.
- ↑ "General Conference on Weights and Measures approves possible changes to the International System of Units, including redefinition of the kilogram" (PDF) (Press release). Sèvres, France: General Conference on Weights and Measures. 23 October 2011. Archived (PDF) from the original on 9 February 2012. Retrieved 25 October 2011.
- ↑ Wood, B. (3–4 November 2014). "Report on the Meeting of the CODATA Task Group on Fundamental Constants". p. 7. http://www.bipm.org/cc/TGFC/Allowed/Minutes/CODATA_Minutes_14-BIPM-public.pdf. "[BIPM director Martin] Milton responded to a question about what would happen if ... the CIPM or the CGPM voted not to move forward with the redefinition of the SI. He responded that he felt that by that time the decision to move forward should be seen as a foregone conclusion."
- ↑ "2018 CODATA Value: Boltzmann constant". The NIST Reference on Constants, Units, and Uncertainty. NIST. 20 May 2019. http://physics.nist.gov/cgi-bin/cuu/Value?k. Retrieved 2019-05-20.
- ↑ 33.0 33.1 "Resolution 1 of the 26th CGPM (2018)". BIPM. https://www.bipm.org/en/committees/cg/cgpm/26-2018/resolution-1.
- ↑ "Updating the definition of the kelvin". International Bureau for Weights and Measures (BIPM). http://www.bipm.org/wg/CCT/TG-SI/Allowed/Documents/Updating_the_definition_of_the_kelvin2.pdf.
- ↑ Newell, D B; Cabiati, F; Fischer, J; Fujii, K; Karshenboim, S G; Margolis, H S; de Mirandés, E; Mohr, P J et al. (29 January 2018). "The CODATA 2017 values of h, e, k, and NA for the revision of the SI". Metrologia 55 (1): L13–L16. doi:10.1088/1681-7575/aa950a. Bibcode: 2018Metro..55L..13N.
- ↑ "Kelvin: Introduction" (in en). 14 May 2018. https://www.nist.gov/si-redefinition/kelvin-introduction.
- ↑ "Definition of KELVIN" (in en). https://www.merriam-webster.com/dictionary/kelvin.
- ↑ CERN English Language Style Guide. CERN. 2022. pp. 64. https://translation-council-support-group.web.cern.ch/sites/default/files/styles/large/CERN%20TM%20English%20language%20style%20guide.pdf.
- ↑ Brady, James E.; Senese, Fred (28 January 2008) (in en). Chemistry, Student Study Guide: The Study of Matter and Its Changes. John Wiley & Sons. p. 15. ISBN 978-0-470-18464-6. https://books.google.com/books?id=zS1EX-e7kPQC&pg=PA15.
- ↑ The Unicode Standard, Version 8.0. Mountain View, CA, USA: The Unicode Consortium. August 2015. ISBN 978-1-936213-10-8. https://www.unicode.org/versions/Unicode8.0.0/ch22.pdf. Retrieved 6 September 2015.
Bibliography
- Bureau International des Poids et Mesures (2019). "The International System of Units (SI) Brochure". International Committee for Weights and Measures. https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-EN.pdf.
External links
 |


