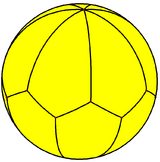
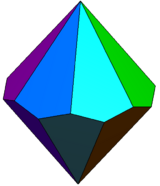
Heptagonal trapezohedron
| Heptagonal trapezohedron | |
|---|---|
| |
| Type | trapezohedron |
| Conway | dA7 |
| Coxeter diagram | |
| Faces | 14 kites |
| Edges | 28 |
| Vertices | 16 |
| Face configuration | V7.3.3.3 |
| Symmetry group | D7d, [2+,14], (2*7), order 28 |
| Rotation group | D7, [2,7]+, (227), order 14 |
| Dual polyhedron | heptagonal antiprism |
| Properties | convex, face-transitive |
In geometry, a heptagonal trapezohedron or deltohedron is the fifth in an infinite series of trapezohedra which are dual polyhedron to the antiprisms. It has 14 faces which are congruent kites.
It is a isohedral figure, (face-transitive), having all its faces the same. More specifically, all faces must be not merely congruent but must be transitive, i.e. must lie within the same symmetry orbit. Convex isohedral polyhedra are the shapes that will make fair dice.[1]
Symmetry
The symmetry a heptagonal trapezohedron is D7d of order 28. The rotation group is D7 of order 14.
Variations
One degree of freedom within symmetry from D7d (order 28) to D7 (order 14) changes the congruent kites into congruent quadrilaterals with three edge lengths, called twisted kites, and the trapezohedron is called a twisted trapezohedron.
If the kites surrounding the two peaks are not twisted but are of two different shapes, the trapezohedron can only have C7v (cyclic) symmetry, order 14, and is called an unequal or asymmetric heptagonal trapezohedron. Its dual is an unequal antiprism, with the top and bottom polygons of different radii. These are still isohedral.
If the kites are twisted and of two different shapes, the trapezohedron can only have C7 (cyclic) symmetry, order 7, and is called an unequal twisted heptagonal trapezohedron.
Spherical tiling
The heptagonal trapezohedron also exists as a spherical tiling, with 2 vertices on the poles, and alternating vertices equally spaced above and below the equator.
See also
References
- ↑ McLean, K. Robin (1990), "Dungeons, dragons, and dice", The Mathematical Gazette 74 (469): 243–256, doi:10.2307/3619822.
External links
- Weisstein, Eric W.. "Trapezohedron". http://mathworld.wolfram.com/Trapezohedron.html.
- Virtual Reality Polyhedra The Encyclopedia of Polyhedra