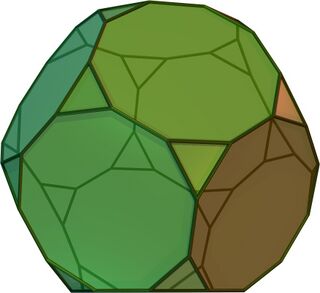
Truncated dodecahedron

Short description: Archimedean solid with 32 faces

Truncated dodecahedron
(Click here for rotating model)
Type	Archimedean solid Uniform polyhedron
Elements	F = 32, E = 90, V = 60 (χ = 2)
Faces by sides	20{3}+12{10}
Conway notation	tD
Schläfli symbols	t{5,3}
	t0,1{5,3}
Wythoff symbol	2 3 | 5
Coxeter diagram
Symmetry group	Ih, H3, [5,3], (*532), order 120
Rotation group	I, [5,3]+, (532), order 60
Dihedral angle	10-10: 116.57° 3-10: 142.62°
References	U26, C29, W10
Properties	Semiregular convex
Colored faces	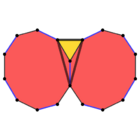3.10.10 (Vertex figure)
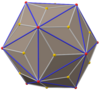Triakis icosahedron (dual polyhedron)	Net

File:Truncated dodecahedron.stl In geometry, the truncated dodecahedron is an Archimedean solid. It has 12 regular decagonal faces, 20 regular triangular faces, 60 vertices and 90 edges.

Geometric relations

This polyhedron can be formed from a regular dodecahedron by truncating (cutting off) the corners so the pentagon faces become decagons and the corners become triangles.

It is used in the cell-transitive hyperbolic space-filling tessellation, the bitruncated icosahedral honeycomb.

Area and volume

The area A and the volume V of a truncated dodecahedron of edge length a are:

[math]\displaystyle{ \begin{align} A &= 5 \left(\sqrt{3}+6\sqrt{5+2\sqrt{5}}\right) a^2 &&\approx 100.990\,76a^2 \\ V &= \tfrac{5}{12} \left(99+47\sqrt{5}\right) a^3 &&\approx 85.039\,6646a^3 \end{align} }[/math]

Cartesian coordinates

Cartesian coordinates for the vertices of a truncated dodecahedron with edge length 2φ − 2, centered at the origin, are all even permutations of:

(0, ±1/φ, ±(2 + φ))

(±1/φ, ±φ, ±2φ)

(±φ, ±2, ±(φ + 1))

where φ = 1 + √5/2 is the golden ratio.

Orthogonal projections

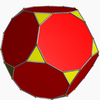
The truncated dodecahedron has five special orthogonal projections, centered: on a vertex, on two types of edges, and two types of faces. The last two correspond to the A₂ and H₂ Coxeter planes.

Orthogonal projections

Centered by	Vertex	Edge 3-3	Edge 10-10	Face Triangle	Face Decagon
Solid
Wireframe
Projective symmetry	[2]	[2]	[2]	[6]	[10]
Dual

Spherical tilings and Schlegel diagrams

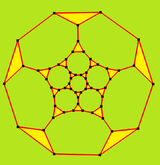
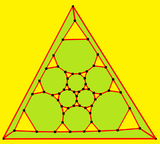
The truncated dodecahedron can also be represented as a spherical tiling, and projected onto the plane via a stereographic projection. This projection is conformal, preserving angles but not areas or lengths. Straight lines on the sphere are projected as circular arcs on the plane.

Schlegel diagrams are similar, with a perspective projection and straight edges.

Orthographic projection	Stereographic projections
	Decagon-centered	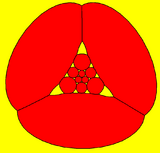Triangle-centered

Vertex arrangement

It shares its vertex arrangement with three nonconvex uniform polyhedra:

Truncated dodecahedron

Great icosicosidodecahedron

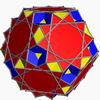Great ditrigonal dodecicosidodecahedron

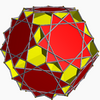Great dodecicosahedron

Related polyhedra and tilings

It is part of a truncation process between a dodecahedron and icosahedron:

This polyhedron is topologically related as a part of sequence of uniform truncated polyhedra with vertex configurations (3.2n.2n), and [n,3] Coxeter group symmetry.

Truncated dodecahedral graph

Truncated dodecahedral graph
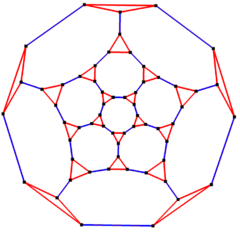5-fold symmetry Schlegel diagram
Vertices	60
Edges	90
Automorphisms	120
Chromatic number	3
Chromatic index	3
Properties	Cubic, Hamiltonian, regular, zero-symmetric
Table of graphs and parameters

In the mathematical field of graph theory, a truncated dodecahedral graph is the graph of vertices and edges of the truncated dodecahedron, one of the Archimedean solids. It has 60 vertices and 90 edges, and is a cubic Archimedean graph.

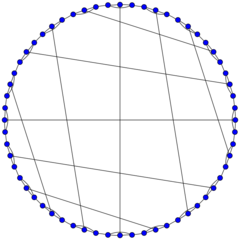Circular

References

• Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.  (Section 3-9)
• Cromwell, P. (1997). Polyhedra. United Kingdom: Cambridge. pp. 79–86 Archimedean solids. ISBN 0-521-55432-2. 

External links

• Eric W. Weisstein, Truncated dodecahedron (Archimedean solid) at MathWorld.
  • Weisstein, Eric W.. "Truncated dodecahedral graph". http://mathworld.wolfram.com/TruncatedDodecahedralGraph.html. 
• Klitzing, Richard. "3D convex uniform polyhedra o3x5x - tid". https://bendwavy.org/klitzing/dimensions/polyhedra.htm. 
• Editable printable net of a truncated dodecahedron with interactive 3D view
• The Uniform Polyhedra
• Virtual Reality Polyhedra The Encyclopedia of Polyhedra