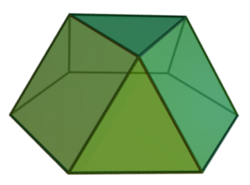
Triangular cupola
| Triangular cupola | |
|---|---|
 | |
| Type | Johnson J2 – J3 – J4 |
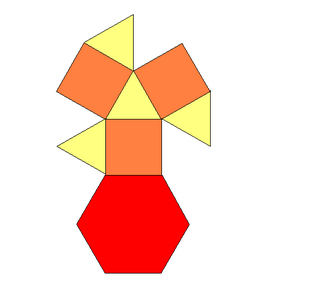
| Faces | 4 triangles 3 squares 1 hexagon |
| Edges | 15 |
| Vertices | 9 |
| Vertex configuration | 6(3.4.6) 3(3.4.3.4) |
| Symmetry group | C3v |
| Dual polyhedron | https://levskaya.github.io/polyhedronisme/?recipe=C1000dJ3 |
| Properties | convex |
| Net | |
 | |
In geometry, the triangular cupola is one of the Johnson solids (J3). It can be seen as half a cuboctahedron.
A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[1]
Formulae
The following formulae for the volume ([math]\displaystyle{ V }[/math]), the surface area ([math]\displaystyle{ A }[/math]) and the height ([math]\displaystyle{ H }[/math]) can be used if all faces are regular, with edge length a:[2][3]
- [math]\displaystyle{ V=\left(\frac{5}{3\sqrt{2}}\right) a^3\approx1.17851...a^3 }[/math]
- [math]\displaystyle{ A=\left(3+\frac{5\sqrt{3}}{2} \right) a^2\approx7.33013...a^2 }[/math]
- [math]\displaystyle{ H = \frac{\sqrt{6}}{3} a\approx 0.816496...a }[/math]
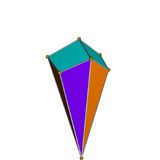
Dual polyhedron
The dual of the triangular cupola has 6 triangular and 3 kite faces:
| Dual triangular cupola | Net of dual |
|---|---|
|

|
Related polyhedra and honeycombs
The triangular cupola can be augmented by 3 square pyramids, leaving adjacent coplanar faces. This isn't a Johnson solid because of its coplanar faces. Merging those coplanar triangles into larger ones, topologically this is another triangular cupola with isosceles trapezoidal side faces. If all the triangles are retained and the base hexagon is replaced by 6 triangles, it generates a coplanar deltahedron with 22 faces.
The triangular cupola can form a tessellation of space with square pyramids and/or octahedra,[4] the same way octahedra and cuboctahedra can fill space.
The family of cupolae with regular polygons exists up to n=5 (pentagons), and higher if isosceles triangles are used in the cupolae.
References
- ↑ Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics 18: 169–200, doi:10.4153/cjm-1966-021-8.
- ↑ Stephen Wolfram, "Triangular cupola" from Wolfram Alpha. Retrieved July 20, 2010.
- ↑ Sapiña, R.. "Area and volume of the Johnson solid J₃" (in es). Problemas y Ecuaciones. ISSN 2659-9899. https://www.problemasyecuaciones.com/geometria3D/volumen/Johnson/J3/calculadora-area-volumen-formulas.html. Retrieved 2020-09-08.
- ↑ "J3 honeycomb". http://woodenpolyhedra.web.fc2.com/J3.html.
External links
 |


