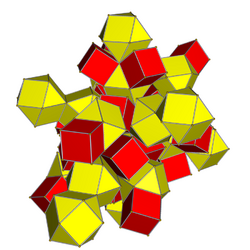
Rectified 24-cell
| Rectified 24-cell | ||
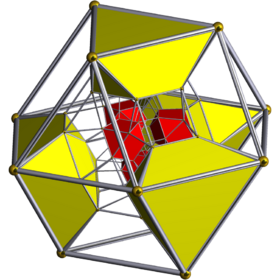 Schlegel diagram 8 of 24 cuboctahedral cells shown | ||
| Type | Uniform 4-polytope | |
| Schläfli symbols | r{3,4,3} = [math]\displaystyle{ \left\{\begin{array}{l}3\\4,3\end{array}\right\} }[/math] rr{3,3,4}=[math]\displaystyle{ r\left\{\begin{array}{l}3\\3,4\end{array}\right\} }[/math] r{31,1,1} = [math]\displaystyle{ r\left\{\begin{array}{l}3\\3\\3\end{array}\right\} }[/math] | |
| Coxeter diagrams | ||
| Cells | 48 | 24 3.4.3.4 24 4.4.4 |
| Faces | 240 | 96 {3} 144 {4} |
| Edges | 288 | |
| Vertices | 96 | |
| Vertex figure |  50px50px 50px50pxTriangular prism | |
| Symmetry groups | F4 [3,4,3], order 1152 B4 [3,3,4], order 384 D4 [31,1,1], order 192 | |
| Properties | convex, edge-transitive | |
| Uniform index | 22 23 24 | |
In geometry, the rectified 24-cell or rectified icositetrachoron is a uniform 4-dimensional polytope (or uniform 4-polytope), which is bounded by 48 cells: 24 cubes, and 24 cuboctahedra. It can be obtained by rectification of the 24-cell, reducing its octahedral cells to cubes and cuboctahedra.[1]
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as tC24.
It can also be considered a cantellated 16-cell with the lower symmetries B4 = [3,3,4]. B4 would lead to a bicoloring of the cuboctahedral cells into 8 and 16 each. It is also called a runcicantellated demitesseract in a D4 symmetry, giving 3 colors of cells, 8 for each.
Construction
The rectified 24-cell can be derived from the 24-cell by the process of rectification: the 24-cell is truncated at the midpoints. The vertices become cubes, while the octahedra become cuboctahedra.
Cartesian coordinates
A rectified 24-cell having an edge length of √2 has vertices given by all permutations and sign permutations of the following Cartesian coordinates:
- (0,1,1,2) [4!/2!×23 = 96 vertices]
The dual configuration with edge length 2 has all coordinate and sign permutations of:
- (0,2,2,2) [4×23 = 32 vertices]
- (1,1,1,3) [4×24 = 64 vertices]
Images
| Stereographic projection | |
|---|---|
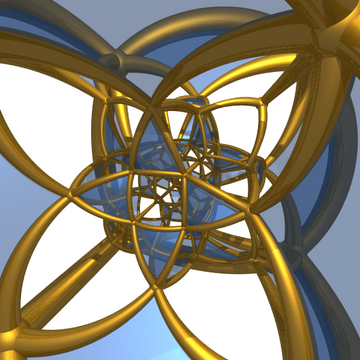 | |
| Center of stereographic projection with 96 triangular faces blue | |
Symmetry constructions
There are three different symmetry constructions of this polytope. The lowest [math]\displaystyle{ {D}_4 }[/math] construction can be doubled into [math]\displaystyle{ {C}_4 }[/math] by adding a mirror that maps the bifurcating nodes onto each other. [math]\displaystyle{ {D}_4 }[/math] can be mapped up to [math]\displaystyle{ {F}_4 }[/math] symmetry by adding two mirror that map all three end nodes together.
The vertex figure is a triangular prism, containing two cubes and three cuboctahedra. The three symmetries can be seen with 3 colored cuboctahedra in the lowest [math]\displaystyle{ {D}_4 }[/math] construction, and two colors (1:2 ratio) in [math]\displaystyle{ {C}_4 }[/math], and all identical cuboctahedra in [math]\displaystyle{ {F}_4 }[/math].
| Coxeter group | [math]\displaystyle{ {F}_4 }[/math] = [3,4,3] | [math]\displaystyle{ {C}_4 }[/math] = [4,3,3] | [math]\displaystyle{ {D}_4 }[/math] = [3,31,1] |
|---|---|---|---|
| Order | 1152 | 384 | 192 |
| Full symmetry group |
[3,4,3] | [4,3,3] | <[3,31,1]> = [4,3,3] [3[31,1,1]] = [3,4,3] |
| Coxeter diagram | |||
| Facets | 3: 2: |
2,2: 2: |
1,1,1: 2: |
| Vertex figure | 
|

|

|
Alternate names
- Rectified 24-cell, Cantellated 16-cell (Norman Johnson)
- Rectified icositetrachoron (Acronym rico) (George Olshevsky, Jonathan Bowers)
- Cantellated hexadecachoron
- Disicositetrachoron
- Amboicositetrachoron (Neil Sloane & John Horton Conway)
Related polytopes
The convex hull of the rectified 24-cell and its dual (assuming that they are congruent) is a nonuniform polychoron composed of 192 cells: 48 cubes, 144 square antiprisms, and 192 vertices. Its vertex figure is a triangular bifrustum.
Related uniform polytopes
The rectified 24-cell can also be derived as a cantellated 16-cell:
Citations
- ↑ Coxeter 1973, p. 154, §8.4.
References
- T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
- Coxeter, H.S.M. (1973). Regular Polytopes (3rd ed.). New York: Dover.
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26. pp. 409: Hemicubes: 1n1)
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
- 2. Convex uniform polychora based on the tesseract (8-cell) and hexadecachoron (16-cell) - Model 23, George Olshevsky.
- 3. Convex uniform polychora based on the icositetrachoron (24-cell) - Model 23, George Olshevsky.
- 7. Uniform polychora derived from glomeric tetrahedron B4 - Model 23, George Olshevsky.
- Klitzing, Richard. "4D uniform polytopes (polychora) o3x4o3o - rico". https://bendwavy.org/klitzing/dimensions/polychora.htm.
Fundamental convex regular and uniform polytopes in dimensions 2–10
| ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Family | An | Bn | I2(p) / Dn | E6 / E7 / E8 / F4 / G2 | Hn | |||||||
| Regular polygon | Triangle | Square | p-gon | Hexagon | Pentagon | |||||||
| Uniform polyhedron | Tetrahedron | Octahedron • Cube | Demicube | Dodecahedron • Icosahedron | ||||||||
| Uniform 4-polytope | 5-cell | 16-cell • Tesseract | Demitesseract | 24-cell | 120-cell • 600-cell | |||||||
| Uniform 5-polytope | 5-simplex | 5-orthoplex • 5-cube | 5-demicube | |||||||||
| Uniform 6-polytope | 6-simplex | 6-orthoplex • 6-cube | 6-demicube | 122 • 221 | ||||||||
| Uniform 7-polytope | 7-simplex | 7-orthoplex • 7-cube | 7-demicube | 132 • 231 • 321 | ||||||||
| Uniform 8-polytope | 8-simplex | 8-orthoplex • 8-cube | 8-demicube | 142 • 241 • 421 | ||||||||
| Uniform 9-polytope | 9-simplex | 9-orthoplex • 9-cube | 9-demicube | |||||||||
| Uniform 10-polytope | 10-simplex | 10-orthoplex • 10-cube | 10-demicube | |||||||||
| Uniform n-polytope | n-simplex | n-orthoplex • n-cube | n-demicube | 1k2 • 2k1 • k21 | n-pentagonal polytope | |||||||
| Topics: Polytope families • Regular polytope • List of regular polytopes and compounds | ||||||||||||
 |