Tetrahexagonal tiling
From HandWiki
In geometry, the tetrahexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol r{6,4}.
Constructions
There are for uniform constructions of this tiling, three of them as constructed by mirror removal from the [6,4] kaleidoscope. Removing the last mirror, [6,4,1+], gives [6,6], (*662). Removing the first mirror [1+,6,4], gives [(4,4,3)], (*443). Removing both mirror as [1+,6,4,1+], leaving [(3,∞,3,∞)] (*3232).
| Uniform Coloring |

|

|

|

|
|---|---|---|---|---|
| Fundamental Domains |

|

|
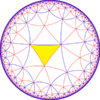
|

|
| Schläfli | r{6,4} | r{4,6}1⁄2 | r{6,4}1⁄2 | r{6,4}1⁄4 |
| Symmetry | [6,4] (*642) |
[6,6] = [6,4,1+] (*662) |
[(4,4,3)] = [1+,6,4] (*443) |
[(∞,3,∞,3)] = [1+,6,4,1+] (*3232) |
| Symbol | r{6,4} | rr{6,6} | r(4,3,4) | t0,1,2,3(∞,3,∞,3) |
| Coxeter diagram |
Symmetry
The dual tiling, called a rhombic tetrahexagonal tiling, with face configuration V4.6.4.6, and represents the fundamental domains of a quadrilateral kaleidoscope, orbifold (*3232), shown here in two different centered views. Adding a 2-fold rotation point in the center of each rhombi represents a (2*32) orbifold.
Related polyhedra and tiling
See also
- Square tiling
- Tilings of regular polygons
- List of uniform planar tilings
- List of regular polytopes
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN:978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8.
External links
- Weisstein, Eric W.. "Hyperbolic tiling". http://mathworld.wolfram.com/HyperbolicTiling.html.
- Weisstein, Eric W.. "Poincaré hyperbolic disk". http://mathworld.wolfram.com/PoincareHyperbolicDisk.html.
- Hyperbolic and Spherical Tiling Gallery
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
- Hyperbolic Planar Tessellations, Don Hatch
 |





