Trihexagonal tiling

From HandWiki
Short description: Tiling of the plane by regular hexagons and equilateral triangles

In geometry, the trihexagonal tiling is one of 11 uniform tilings of the Euclidean plane by regular polygons.[1] It consists of equilateral triangles and regular hexagons, arranged so that each hexagon is surrounded by triangles and vice versa. The name derives from the fact that it combines a regular hexagonal tiling and a regular triangular tiling. Two hexagons and two triangles alternate around each vertex, and its edges form an infinite arrangement of lines. Its dual is the rhombille tiling.[2]

This pattern, and its place in the classification of uniform tilings, was already known to Johannes Kepler in his 1619 book Harmonices Mundi.[3] The pattern has long been used in Japanese basketry, where it is called kagome. The Japanese term for this pattern has been taken up in physics, where it is called a kagome lattice. It occurs also in the crystal structures of certain minerals. Conway calls it a hexadeltille, combining alternate elements from a hexagonal tiling (hextille) and triangular tiling (deltille).[4]

Kagome

Japanese basket showing the kagome pattern

Kagome (Japanese: 籠目) is a traditional Japanese woven bamboo pattern; its name is composed from the words kago, meaning "basket", and me, meaning "eye(s)", referring to the pattern of holes in a woven basket.

The kagome pattern is common in bamboo weaving in East Asia. In 2022, archaeologists found bamboo weaving remains at the Dongsunba ruins in Chongqing, China, 200 BC. After 2200 years, the kagome pattern is still clear.[5][6]

  • Inuit snowshoe Tagluk

  • Kagome pattern in detail

It is a woven arrangement of laths composed of interlaced triangles such that each point where two laths cross has four neighboring points, forming the pattern of a trihexagonal tiling. The woven process gives the Kagome a chiral wallpaper group symmetry, p6 (632).

Kagome lattice

The term kagome lattice was coined by Japanese physicist Kôdi Husimi, and first appeared in a 1951 paper by his assistant Ichirō Shōji.[7] The kagome lattice in this sense consists of the vertices and edges of the trihexagonal tiling. Despite the name, these crossing points do not form a mathematical lattice.

A related three dimensional structure formed by the vertices and edges of the quarter cubic honeycomb, filling space by regular tetrahedra and truncated tetrahedra, has been called a hyper-kagome lattice.[8] It is represented by the vertices and edges of the quarter cubic honeycomb, filling space by regular tetrahedra and truncated tetrahedra. It contains four sets of parallel planes of points and lines, each plane being a two dimensional kagome lattice. A second expression in three dimensions has parallel layers of two dimensional lattices and is called an orthorhombic-kagome lattice.[8] The trihexagonal prismatic honeycomb represents its edges and vertices.

Some minerals, namely jarosites and herbertsmithite, contain two-dimensional layers or three-dimensional kagome lattice arrangement of atoms in their crystal structure. These minerals display novel physical properties connected with geometrically frustrated magnetism. For instance, the spin arrangement of the magnetic ions in Co3V2O8 rests in a kagome lattice which exhibits fascinating magnetic behavior at low temperatures.[9] Quantum magnets realized on Kagome metals have been discovered to exhibit many unexpected electronic and magnetic phenomena.[10][11][12][13] It is also proposed that SYK behavior can be observed in two dimensional kagome lattice with impurities.[14]

The term is much in use nowadays in the scientific literature, especially by theorists studying the magnetic properties of a theoretical kagome lattice.

See also: Kagome crests.

Symmetry

30-60-90 triangle fundamental domains of p6m (*632) symmetry

The trihexagonal tiling has Schläfli symbol of r{6,3}, or Coxeter diagram, CDel node.pngCDel 6.pngCDel node 1.pngCDel 3.pngCDel node.png, symbolizing the fact that it is a rectified hexagonal tiling, {6,3}. Its symmetries can be described by the wallpaper group p6mm, (*632),[15] and the tiling can be derived as a Wythoff construction within the reflectional fundamental domains of this group. The trihexagonal tiling is a quasiregular tiling, alternating two types of polygons, with vertex configuration (3.6)2. It is also a uniform tiling, one of eight derived from the regular hexagonal tiling.

Uniform colorings

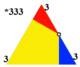
There are two distinct uniform colorings of a trihexagonal tiling. Naming the colors by indices on the 4 faces around a vertex (3.6.3.6): 1212, 1232.[1] The second is called a cantic hexagonal tiling, h2{6,3}, with two colors of triangles, existing in p3m1 (*333) symmetry.

Symmetry p6m, (*632) p3m, (*333)
Coloring Uniform polyhedron-63-t1.png Uniform tiling 333-t12.png
fundamental
domain 		632 fundamental domain t1.png 333 fundamental domain t01.png
Wythoff 2 | 6 3 3 3 | 3
Coxeter CDel node.pngCDel 6.pngCDel node 1.pngCDel 3.pngCDel node.png CDel branch 10ru.pngCDel split2.pngCDel node 1.png = CDel node h1.pngCDel 6.pngCDel node.pngCDel 3.pngCDel node 1.png
Schläfli r{6,3} r{3[3]} = h2{6,3}

Circle packing

The trihexagonal tiling can be used as a circle packing, placing equal diameter circles at the center of every point.[16] Every circle is in contact with 4 other circles in the packing (kissing number).

1-uniform-7-circlepack.svg

Topologically equivalent tilings

The trihexagonal tiling can be geometrically distorted into topologically equivalent tilings of lower symmetry.[1] In these variants of the tiling, the edges do not necessarily line up to form straight lines.

p3m1, (*333) p3, (333) p31m, (3*3) cmm, (2*22)
Trihexagonal tiling unequal.png 3-9-star-tiling.png Hex-hexstar-tiling.svg Trihexagonal tiling unequal2.svg Distorted trihexagonal tiling.png Triangle and triangular star tiling.png Trihexagonal tiling in square tiling.svg

Related quasiregular tilings

The trihexagonal tiling exists in a sequence of symmetries of quasiregular tilings with vertex configurations (3.n)2, progressing from tilings of the sphere to the Euclidean plane and into the hyperbolic plane. With orbifold notation symmetry of *n32 all of these tilings are wythoff construction within a fundamental domain of symmetry, with generator points at the right angle corner of the domain.[17][18]

Related regular complex apeirogons

There are 2 regular complex apeirogons, sharing the vertices of the trihexagonal tiling. Regular complex apeirogons have vertices and edges, where edges can contain 2 or more vertices. Regular apeirogons p{q}r are constrained by: 1/p + 2/q + 1/r = 1. Edges have p vertices arranged like a regular polygon, and vertex figures are r-gonal.[19]

The first is made of triangular edges, two around every vertex, second has hexagonal edges, two around every vertex.

Complex apeirogon 3-12-2.png Complex apeirogon 6-6-2.png
3{12}2 or CDel 3node 1.pngCDel 12.pngCDel node.png 6{6}2 or CDel 6node 1.pngCDel 6.pngCDel node.png

See also

References

  1. ↑ 1.0 1.1 1.2 Grünbaum, Branko; Shephard, G. C. (1987). Tilings and Patterns. W. H. Freeman. ISBN 978-0-7167-1193-3. https://archive.org/details/isbn_0716711931.  See in particular Theorem 2.1.3, p. 59 (classification of uniform tilings); Figure 2.1.5, p.63 (illustration of this tiling), Theorem 2.9.1, p. 103 (classification of colored tilings), Figure 2.9.2, p. 105 (illustration of colored tilings), Figure 2.5.3(d), p. 83 (topologically equivalent star tiling), and Exercise 4.1.3, p. 171 (topological equivalence of trihexagonal and two-triangle tilings).
  2. ↑ Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. p. 38. ISBN 0-486-23729-X. 
  3. ↑ Aiton, E. J.; Duncan, Alistair Matheson; Field, Judith Veronica, eds (1997). The Harmony of the World by Johannes Kepler. Memoirs of the American Philosophical Society. 209. American Philosophical Society. pp. 104–105. ISBN 978-0-87169-209-2. https://books.google.com/books?id=rEkLAAAAIAAJ&pg=PA104. .
  4. ↑ "Chapter 21: Naming Archimedean and Catalan polyhedra and tilings; Euclidean plane tessellations". The Symmetries of Things. Wellesley, MA: A K Peters, Ltd.. 2008. p. 288. ISBN 978-1-56881-220-5. 
  5. ↑ China Central Television, CCTV-13 News Channel (2022-03-25). "[News Live Room Bamboo weaving products of Ba culture first appeared in Chongqing about 2200 years ago"]. https://tv.cctv.com/2022/03/25/VIDERnzB5HyTqn1IMNTHCtnL220325.shtml. 
  6. ↑ Yin, Jia-Xin (March 2023). "Exploring hitherto unknown quantum phases in kagome crystals". Physics (物理) 52 (3): 157–165. doi:10.7693/wl20230301. 
  7. ↑ Mekata, Mamoru (February 2003). "Kagome: The story of the basketweave lattice". Physics Today 56 (2): 12–13. doi:10.1063/1.1564329. Bibcode: 2003PhT....56b..12M. 
  8. ↑ 8.0 8.1 Lawler, Michael J.; Kee, Hae-Young; Kim, Yong Baek; Vishwanath, Ashvin (2008). "Topological spin liquid on the hyperkagome lattice of Na4Ir3O8". Physical Review Letters 100 (22): 227201. doi:10.1103/physrevlett.100.227201. PMID 18643453. Bibcode: 2008PhRvL.100v7201L. 
  9. ↑ Yen, F.; Chaudhury, R. P.; Galstyan, E.; Lorenz, B.; Wang, Y. Q.; Sun, Y. Y.; Chu, C. W. (2008). "Magnetic phase diagrams of the Kagome staircase compound Co3V2O8". Physica B: Condensed Matter 403 (5–9): 1487–1489. doi:10.1016/j.physb.2007.10.334. Bibcode: 2008PhyB..403.1487Y. 
  10. ↑ "A quantum magnet with a topological twist" (in en-US). 2019-02-22. https://discovery.princeton.edu/2019/02/22/a-quantum-magnet-with-a-topological-twist/. 
  11. ↑ Yin, Jia-Xin; Zhang, Songtian S.; Li, Hang; Jiang, Kun; Chang, Guoqing; Zhang, Bingjing; Lian, Biao; Xiang, Cheng et al. (2018). "Giant and anisotropic many-body spin–orbit tunability in a strongly correlated kagome magnet". Nature 562 (7725): 91–95. doi:10.1038/s41586-018-0502-7. PMID 30209398. Bibcode: 2018Natur.562...91Y. https://www.nature.com/articles/s41586-018-0502-7. 
  12. ↑ Yin, Jia-Xin; Zhang, Songtian S.; Chang, Guoqing; Wang, Qi; Tsirkin, Stepan S.; Guguchia, Zurab; Lian, Biao; Zhou, Huibin et al. (2019). "Negative flat band magnetism in a spin–orbit-coupled correlated kagome magnet". Nature Physics 15 (5): 443–8. doi:10.1038/s41567-019-0426-7. Bibcode: 2019NatPh..15..443Y. https://www.nature.com/articles/s41567-019-0426-7. 
  13. ↑ Yazyev, Oleg V. (2019). "An upside-down magnet". Nature Physics 15 (5): 424–5. doi:10.1038/s41567-019-0451-6. Bibcode: 2019NatPh..15..424Y. https://www.nature.com/articles/s41567-019-0451-6. 
  14. ↑ Wei, Chenan; Sedrakyan, Tigran (2021-01-29). "Optical lattice platform for the Sachdev-Ye-Kitaev model". Phys. Rev. A 103 (1): 013323. doi:10.1103/PhysRevA.103.013323. Bibcode: 2021PhRvA.103a3323W. 
  15. ↑ Steurer, Walter; Deloudi, Sofia (2009). Crystallography of Quasicrystals: Concepts, Methods and Structures. Springer Series in Materials Science. 126. Springer. p. 20. ISBN 978-3-642-01899-2. https://books.google.com/books?id=nVx-tu596twC&pg=PA20. 
  16. ↑ Critchlow, Keith (2000). "pattern G". Order in Space: A design source book. Thames & Hudson. pp. 74–75. ISBN 978-0-500-34033-2. 
  17. ↑ Coxeter, H.S.M. (1973). "V. The Kaleidoscope, §5.7 Wythoff's construction". Regular Polytopes (3rd ed.). Dover. ISBN 0-486-61480-8. 
  18. ↑ Huson, Daniel H. "Two Dimensional symmetry Mutations". CiteSeerX 10.1.1.30.8536.
  19. ↑ Coxeter, H.S.M. (1991). Regular Complex Polytopes (2nd ed.). Cambridge University Press. pp. 111–2, 136. ISBN 978-0-521-39490-1. 

Further reading

  • Seymour, Dale; Britton, Jill (1989). Introduction to Tessellations. pp. 50–56. ISBN 978-0-86651-461-3. 



Retrieved from "https://handwiki.org/wiki/index.php?title=Trihexagonal_tiling&oldid=3720261"