Einstein problem

Short description: Question about single-shape aperiodic tiling

Aperiodic tiling with "Tile(1,1)". The tiles are colored according to their rotational orientation modulo 60 degrees. (Smith, Myers, Kaplan, and Goodman-Strauss)

In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; that is, a shape that can tessellate space but only in a nonperiodic way. Such a shape is called an einstein, a word play on ein Stein, German for "one stone".

Several variants of the problem, depending on the particular definitions of nonperiodicity and the specifications of what sets may qualify as tiles and what types of matching rules are permitted, were solved beginning in the 1990s. The strictest version of the problem was solved in 2023 (the initial discovery was in November 2022, with a preprint published in March 2023), pending peer review.

The einstein problem can be seen as a natural extension of the second part of Hilbert's eighteenth problem, which asks for a single polyhedron that tiles Euclidean 3-space, but such that no tessellation by this polyhedron is isohedral. Such anisohedral tiles were found by Karl Reinhardt in 1928, but these anisohedral tiles all tile space periodically.

Proposed solutions

In 1988, Peter Schmitt discovered a single aperiodic prototile in three-dimensional Euclidean space. While no tiling by this prototile admits a translation as a symmetry, some have a screw symmetry. The screw operation involves a combination of a translation and a rotation through an irrational multiple of π, so no number of repeated operations ever yield a pure translation. This construction was subsequently extended by John Horton Conway and Ludwig Danzer to a convex aperiodic prototile, the Schmitt–Conway–Danzer tile. The presence of the screw symmetry resulted in a reevaluation of the requirements for non-periodicity. Chaim Goodman-Strauss suggested that a tiling be considered strongly aperiodic if it admits no infinite cyclic group of Euclidean motions as symmetries, and that only tile sets which enforce strong aperiodicity be called strongly aperiodic, while other sets are to be called weakly aperiodic.

The Socolar–Taylor tile was proposed in 2010 as a solution to the einstein problem, but this tile is not a connected set.

In 1996, Petra Gummelt constructed a decorated decagonal tile and showed that when two kinds of overlaps between pairs of tiles are allowed, the tiles can cover the plane, but only non-periodically. A tiling is usually understood to be a covering with no overlaps, and so the Gummelt tile is not considered an aperiodic prototile. An aperiodic tile set in the Euclidean plane that consists of just one tile–the Socolar–Taylor tile–was proposed in early 2010 by Joshua Socolar and Joan Taylor. This construction requires matching rules, rules that restrict the relative orientation of two tiles and that make reference to decorations drawn on the tiles, and these rules apply to pairs of nonadjacent tiles. Alternatively, an undecorated tile with no matching rules may be constructed, but the tile is not connected. The construction can be extended to a three-dimensional, connected tile with no matching rules, but this tile allows tilings that are periodic in one direction, and so it is only weakly aperiodic. Moreover, the tile is not simply connected.

The Hat

The tiling discovered by David Smith

One of the infinite family of Smith–Myers–Kaplan–Goodman-Strauss tiles. Yellow tiles are the reflected versions of the blue tiles.

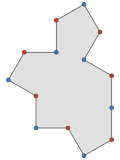
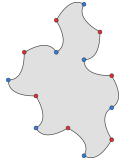
In November 2022, hobbyist David Smith discovered a "hat"-shaped tile formed from eight copies of a 60°–90°–120°–90° kite, glued edge-to-edge, which seemed to only tile the plane aperiodically. Smith recruited help from mathematicians Craig S. Kaplan, Joseph Samuel Myers, and Chaim Goodman-Strauss, and in March 2023 the group posted a preprint proving that the hat, when considered with its mirror image, forms an aperiodic prototile set. Furthermore, the hat can be generalized to an infinite family of tiles with the same aperiodic property. Their proof awaits peer review and formal publication.

Tile(1,1) from Smith, Myers, Kaplan & Goodmann-Strauss on the left. A spectre is obtained by modifying the edges of this polygon as in the middle and right example.

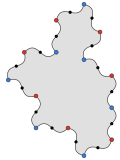
In May 2023 the same team (Smith, Myers, Kaplan, and Goodman-Strauss) posted a new preprint about a family of shapes, called "spectres" and related to the "hat", each of which can tile the plane using only rotations and translations. Furthermore, the "spectre" tile is a "strictly chiral" aperiodic monotile: even if reflections are allowed, every tiling is non-periodic and uses only one chirality of the spectre. That is, there are no tilings of the plane that use both the spectre and its mirror image.

In 2023, a public contest run by the National Museum of Mathematics in New York City and the United Kingdom Mathematics Trust in London asked people to submit creative renditions of the Hat einstein. Out of over 245 submissions from 32 countries, three winners were chosen and received awards at a ceremony at the House of Commons.

See also

• Binary tiling, a weakly aperiodic tiling of the hyperbolic plane with a single tile
• Schmitt–Conway–Danzer tile, in three dimensions

External links

• An aperiodic monotile by Smith, Myers, Kaplan, and Goodman-Strauss
• Haran, Brady; MacDonald, Ayliean (2023). "A New Tile in Newtyle" (video). Numberphile.